PDF
Citation
Print
Introduction
Teeth are known to be one of the strongest and most durable structures in the human body. They are unique to an individual as the fingerprints [1]. Teeth show various pathoses and undergo different types of therapy, which enables them to record information throughout life and even after death [2]. Thus, human dentition provides an important clue in tracing the unknown in forensic odontology. Age estimation along with sex assessment, stature prediction and population designation, assists in reconstructive identification. Dental age estimation has been done using various methods like Gustafson, Demirijan, Willems, Nolla and adopted Havikko methods. It has also been done by the use of radiographs, measuring cementum annulations, root dentinal translucency and even enamel shade color [3-5]. Studies have been conducted which compared the conventional method of measuring the dentin translucency and the digital method of measuring dentin translucency among various age groups [3, 6-8]. In more recent times, it has become well established that digital methods yield superior results in evaluating the translucency length and area than conventional methods. Thus, this study proposes to study the manually ground sections of single rooted teeth using a commercially available image edition software (Adobe Photoshop CC; Adobe). The study also proposes to measure region of interest (ROI) of root translucency zone in “pixel value” (which will be automatically derived by the software) in addition to the root dentinal translucency as a distinct marker to corroborate dental age with chronological age of the subjects stratified under different age groups and to derive a group specific multiple regression formula.
Materials and Methods
The study was approved by the Ethical Committee and the Institutional Review Board (IRB) of S.R.M University (SRMDC/IRB/2015/MDS/No.602 dated 31/10/2017).
Sample size estimation
The sample size was 120 and the sample size was calculated using the G*Power software. The study included 120 single rooted permanent teeth from 120 different patients that had been extracted for orthodontic and periodontal problems. All teeth were examined for the existence of pathological abnormalities that could seriously impair age estimation prior to sectioning [9]. Teeth that were multirooted or with any pathology were excluded from the study. The total number of groups was 6 groups: 11–20, 21–30, 31–40, 41–50, 51–60, and 61–70 years. There were 20 patients per group. In groups 1–6, the numbers of males were 8, 10, 9, 13, 11, and 9 respectively and those of females were 12, 10, 11, 7, 9, and 11 respectively.
Armamentarium
Ground sections were made using micro motor with carborundum disc, Arkansas stone and measured using digital vernier calipers. Measurement of translucency was done using computer hardware, Core i7. 4 GHz CPU with 32 GB RAM and 17-inch CRT monitor, flat-bed scanner, Konica Minolta bizhub 552 (Konica Minolta) and image edition software, Adobe Photoshop CC.
Methodology
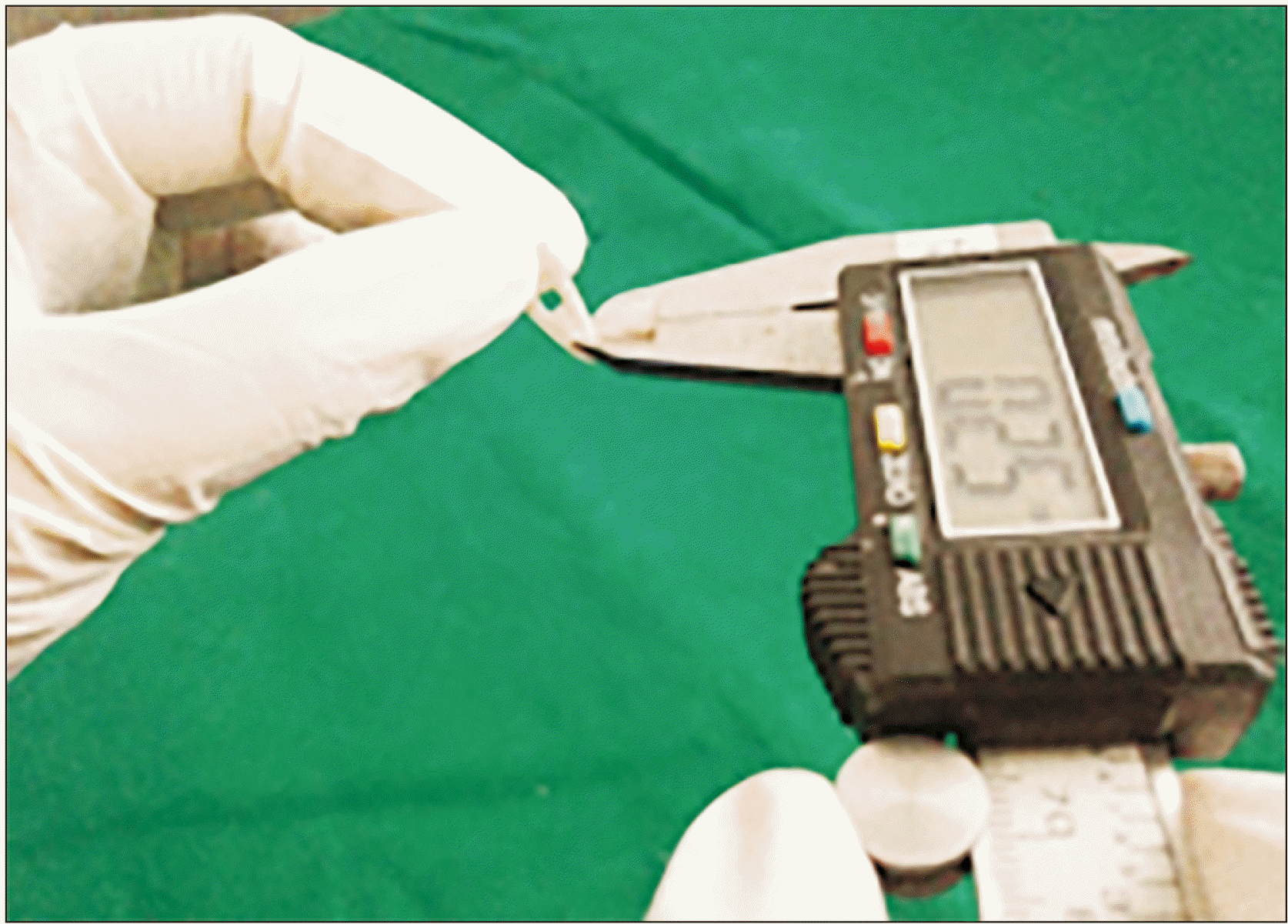
Manual sectioning of teeth was done with rough carborundum stone till a section of 2–3 mm was obtained and further with Arkansas stone to a thickness of 0.25 mm (250 µm). The mean thickness of the section was obtained by measuring the thickness of root dentin at 6 different points (2 points on either side of root canal, at apical, middle and cervical third of root dentin) using a 6” digital caliper that has an accuracy of ±0.001 mm (Fig. 1).
Only the radicular portion of dentin was measured for calculating the area in pixels and for subsequent assessment of translucency length percentage (TLP) and percentage of ROI in pixels (TPP).
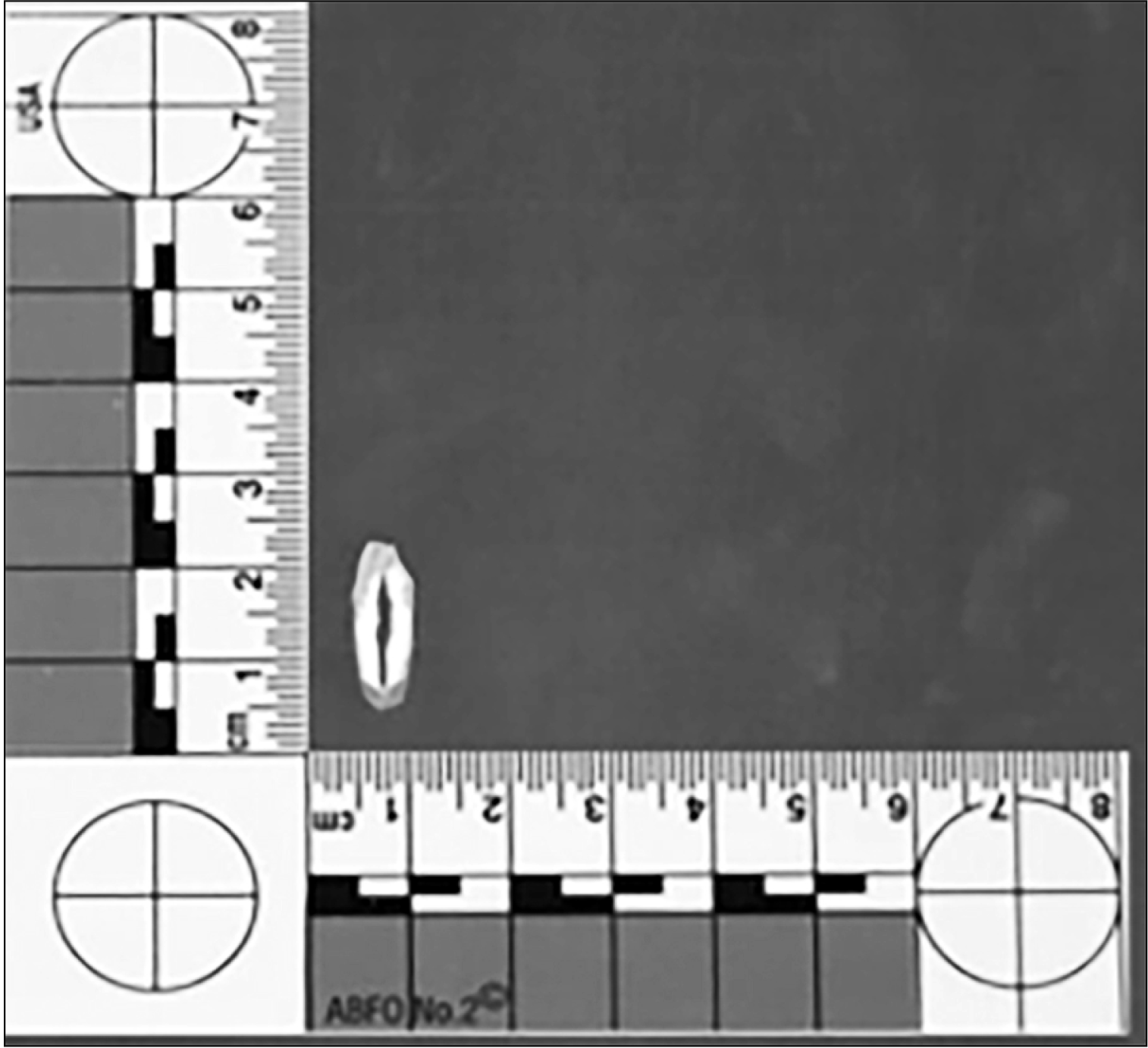
Then, the sections were scanned on a flat-bed scanner. While calculations were made from the scanned image, the resolution of the display (computer monitor) was maintained at 600 dpi to avoid any change in counting of the pixel value of the image (Fig. 2). The following measurements were made.
Measurements
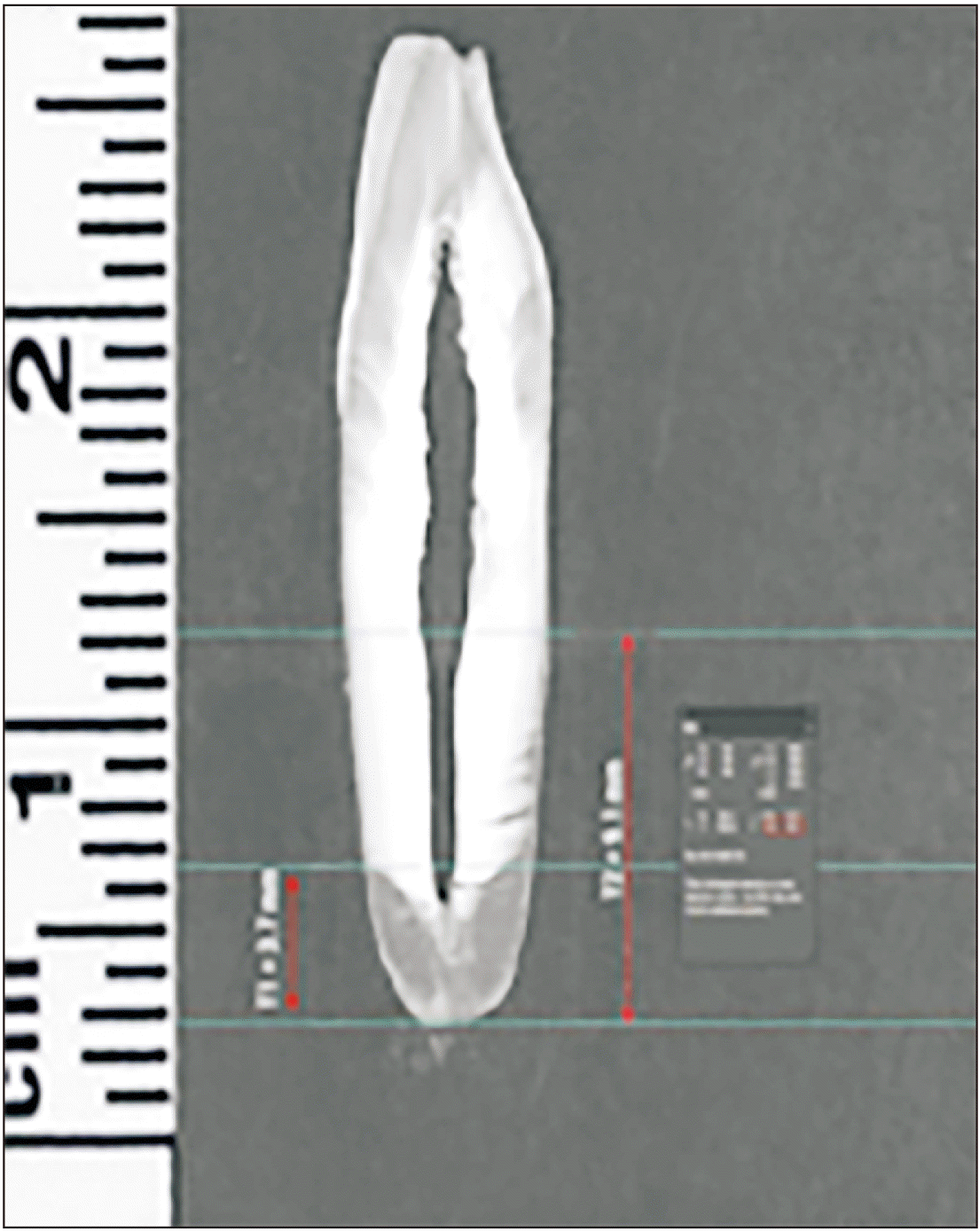
The following measurements were made: 1) root dentin translucency, 2) root length and translucency length (Figs. 3, 4).
Measuring translucency length
To measure the translucency length, the “grid lines” were placed at the apical and coronal extent of translucency and length between the “grid lines” were measured using Photoshop’s in-built measure tool. It was noted that the junction between translucent and non-translucent zones on the labial/buccal and lingual sides in some teeth were depicted a relatively even horizontal line. However, this was not always the case. If this was not so, Bang and Ramm [10] recommend separate measurements of translucency on the two sides of the root canal. This necessitated placement of two guides to represent the labial/buccal and lingual aspects of the coronal extent of translucency separately (T1 and T2 respectively) (Fig. 4). The distance between the coronal and apical guide on each side were measured separately and their average taken. On obtaining the root length and the translucency length, the TLP was calculated using the formula:
TLP=(translucency length/root length)×100.
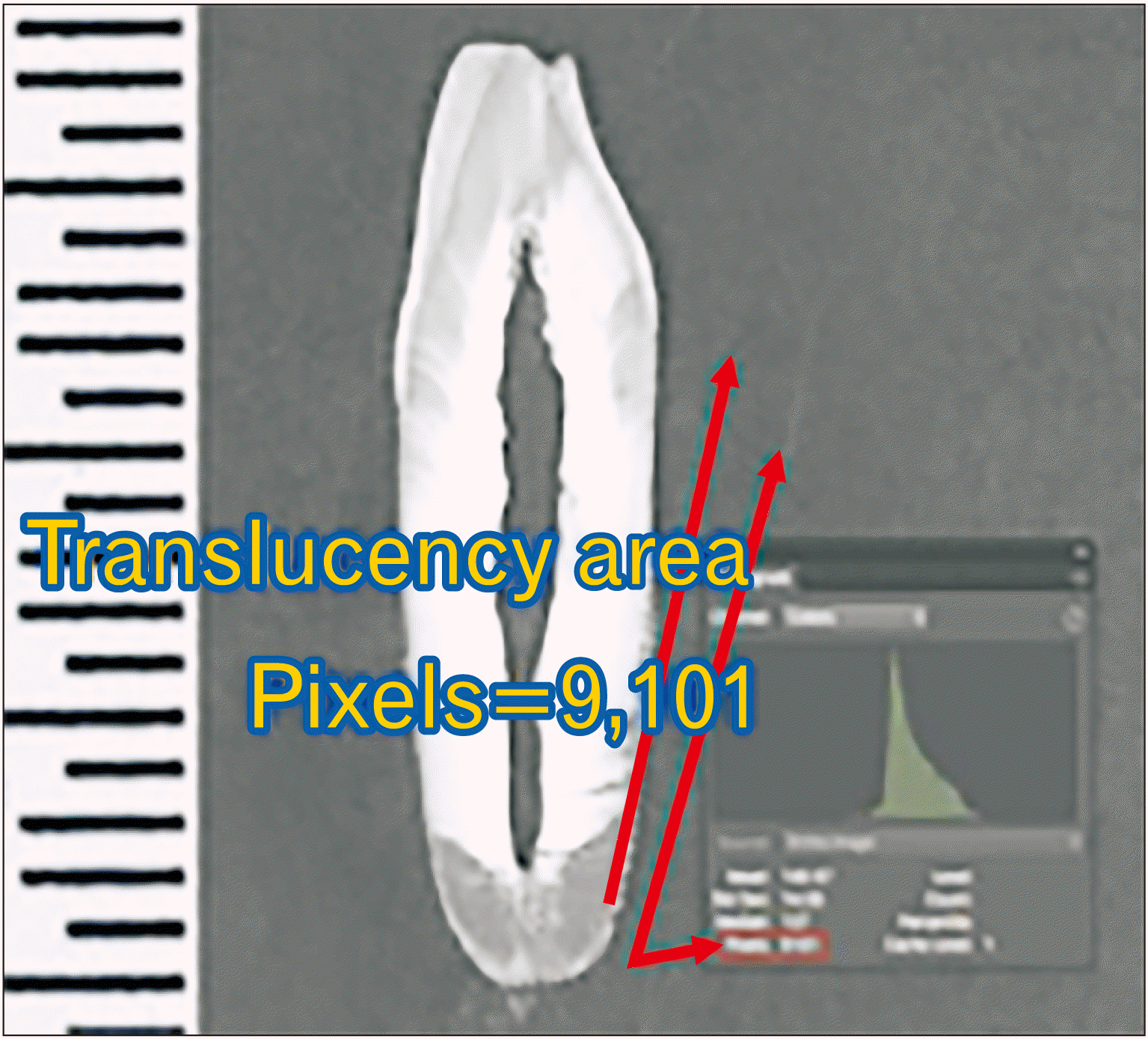
3) Measuring root area in pixels, 4) measuring region of interest of translucency zone (ROI) (Fig. 5) [4] the formula used was:
TPP=(translucency in pixels/root area in pixels)×100.
Statistical analysis
Pearson’s correlation coefficients were obtained for age with TLP and age with TPP. The correlation coefficient (R=0.7221) denotes the degree of correlation between age and TLP and the correlation coefficient (R=0.7848) denoting the degree of correlation between age and TPP was derived. Both these values were statistically significant with regards to age and the P-value being <0.0001. The TPP seemed to have a better correlation with age than that of TLP (Table 1). The correlation coefficient (R) for TLP and TPP was 0.8088, showing a positive correlation between age and both the parameters, TLP and TPP. The coefficient of determination (R2) for TLP and TPP was 0.6541, which was comparatively higher than the values obtained for each individually, i.e., 0.5214 and 0.6159 for TLP and TPP respectively.
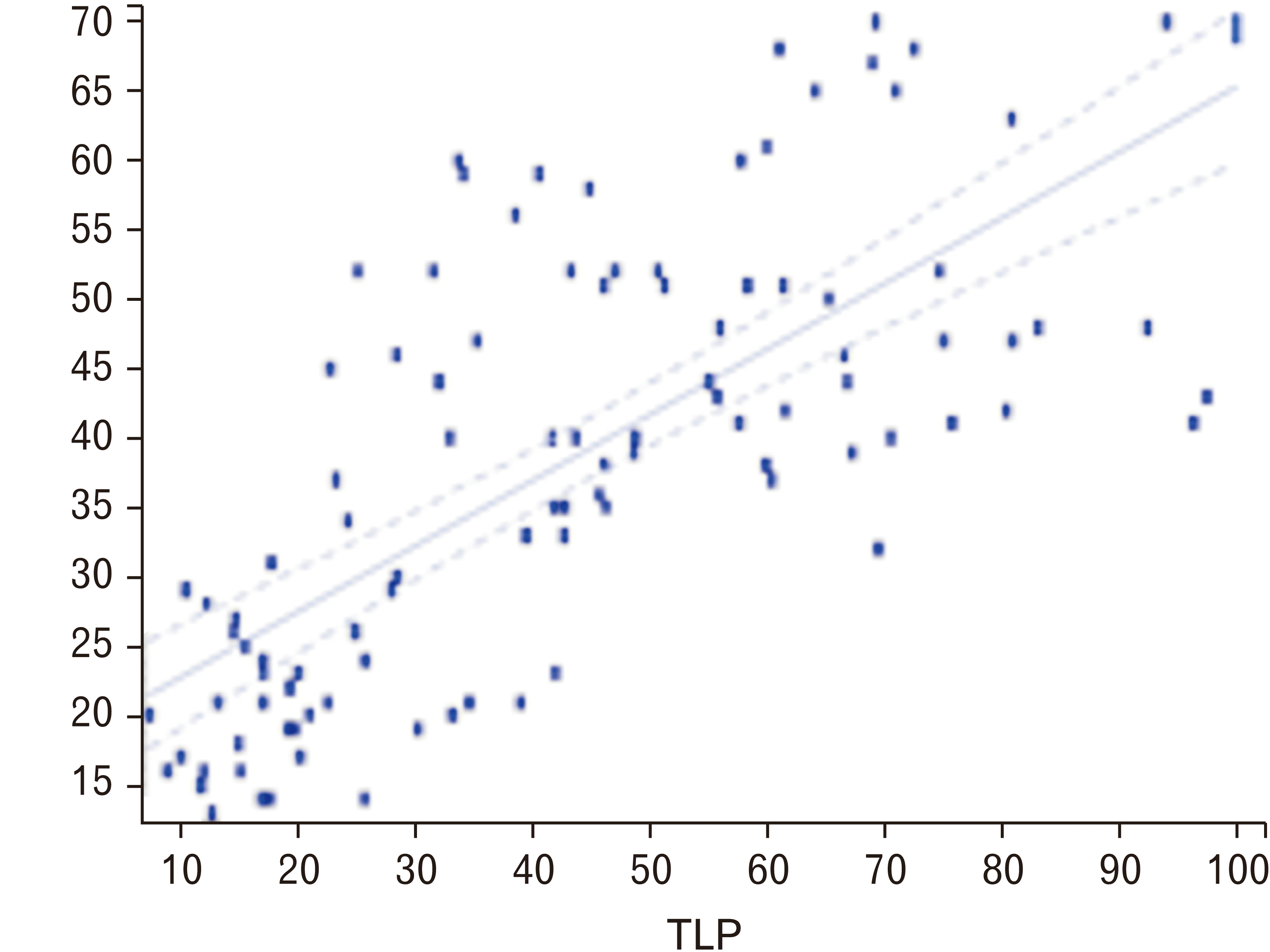
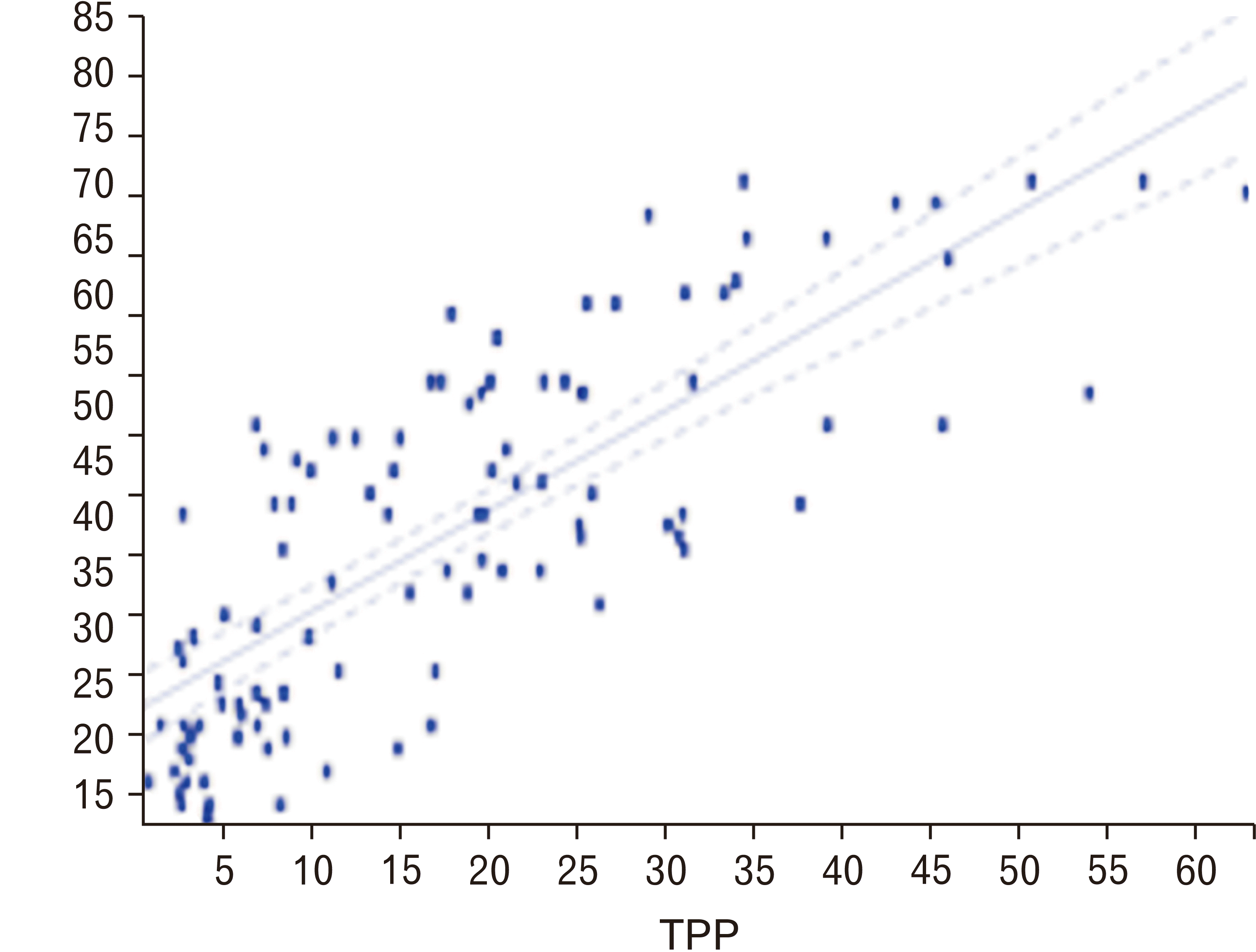
Linear regression equation for 103 samples (y=b+aX), where y is the estimated age, X is the TLP and a and b are constants, was derived individually for TLP (Fig. 6). Linear regression equation for 103 samples (y=b+aZ, where y is the estimated age, Z is the percentage of ROI and a and b are constants) was derived for TPP (Fig. 7).
Results
Out of 120 teeth, 17 teeth were considered outliers and were therefore eliminated before statistical analysis. The correlation coefficient (R=0.8088) between age and both the variables, TLP and TPP, were comparatively higher than the values obtained for TLP and TPP individually. Multilinear regression analysis was done to estimate the age by both these parameters. To achieve the objective of the study, which was to estimate the age of an unknown sample preferably within ±5 years, we further sub-divided the samples into groups based on 30, 20, and 10-year intervals. For each of these groups multilinear regression equations were derived. A step-down analysis was done for each sample, where the estimated age and difference in age was obtained using the appropriate multilinear regression equation from each group. Following this, the mean absolute deviation (MAD) was calculated for each group. It was observed that by using the step-down analysis, the MAD had slightly decreased from 7.945 (MAD from equation 1) to 6.336 (obtained from the appropriate 10-year interval equation, 4A to 4F). By using the step-down analysis method, it was observed that the estimated age obtained was between 0 to 5 years within the actual age in 57/103 (55.33%) of the samples, between 5.1 to 10 years within the actual age in 14/103 (13.59%) of the samples, between 10.1 to 15 years within the actual age in 12/103 (11.65%) of the samples, between 15.1 to 20 years within the actual age in 10/103 (9.7%) of the samples and more than 20 years in 10/103 (9.7%) of the samples. Post-hoc analysis was finally done.
Discussion
This study evaluated the manually ground sections of single rooted teeth using a commercially available image edition software and measured the ROI of root translucency zone in “pixel value” as possible tools for age estimation. Many studies have been previously conducted, with each author providing different equations to estimate the dental age of the subject [3, 4, 7, 10-13]. However, these studies did not give any specific formulae to estimate the age for any particular age group.
Measuring translucency area and root area in pixels rather than in square millimeters
According to Thomas et al. [14], because translucent dentin is not deposited uniformly, its three-dimensional (3D) volume may be more accurate in age estimation. In many sample sections (especially of the younger individuals) it was observed that the root dentin translucency commenced at the root apex and was prominently progressing as a strip of translucency only along the peripheral root surface with no involvement in the body of the root dentin. On the other hand, in older individuals, the sections showed relatively uniform involvement of translucency along the periphery and body of the dentin extending up to the root canal on either side of root canal. Measuring the area of translucency (in square mm) in the traditional way especially in the former sections is slightly complicated while the same derivation in the older individuals would be easier and repeatable. It is simple to only derive both the total root dentin area and the ROI in pixel values (both of which are automatically displayed) [4]. The TLP and TPP were thus used.
Deriving a step-down method by utilizing multilinear regressive equation
Pearson’s correlation coefficient (R) for age and TLP was 0.7221. The coefficient of determination (R2) was 0.5214 (Table 1). The correlation coefficient (R) for age and TPP was 0.7848. The coefficient of determination (R2) was 0.6159 (Table 1). Both these values were statistically significant, with TPP having a better correlation with age than that of TLP with age. The correlation coefficient (R) was 0.8088 and coefficient of determination (R2) was 0.6541, which showed a strong positive correlation between age and both the parameters (length and area), indicating that there is a definite increase in the TLP and TPP with increase in the age (Table 1) prompting the use of a multilinear regression equation. Dalitz [15] used multiple linear regression analysis and advocated in estimating age using multiple parameters. This is practiced under the assumption that different age-related changes have variable correlation to age [16]. After obtaining the correlation coefficient, multilinear regression equation 1 was derived for the 103 samples (age 11–70) and the equation obtained was:
Age=18.481+0.1956*[TLP]+0.6427*[TPP]. The Y intercept was 18.48, which meant that the minimum age at which the TPP and the TLP was 0 is 18.48 years. The coefficient of slope for the TLP was 0.1956 and the coefficient of slope for TPP was 0. 6427. Hence, when age advances by a year, there is a 0.001956 mm increase in the TLP and a 0.006427 increase in TPP (Table 2). The samples were sub-divided into a group of 30, 20, and 10-year intervals. Multilinear regression equations for each such sub-divided group were derived.
Why mean absolute deviation?
The absolute value was obtained and the MAD of the group was calculated (as mentioned in results), which was 7.945. This meant that the equation was able to estimate the age of the individual within ±7.9 years of the actual age of the individual. Calculating MAD or difference is more appropriate in real-life situations, produces lower error rates, and is easier to understand [17].
MAD produced by multilinear regression equations including both length and pixel area measurement may also justify its categorization as a moderately good method as defined by Schmeling et al. [18] Solheim and Sundnes [19] have categorized MAD ≤10 years as “acceptable” and designated MAD of ≥15 years “unsatisfactory” in forensic age estimation. After applying either 2A or 2B, the MAD for the 2 groups: 11–40 and 41–70 years were 7.678 and 7.744 respectively.
The sample was sub-divided into 3 groups (20-year interval), namely, 11–30 years, 31–50 years and 51–70 years. Multilinear regression equations were obtained for samples belonging to each of these groups (Table 2).
Based on the estimated age which was obtained using the multiple regression equations 3A, 3B, or 3C, the MAD for the 3 groups 11–30, 31–50, and 51–70 years were 7.732, 2.353, and 9.435, respectively. A slight decrease in the MAD was seen in the group 31–50 years, by using these equations following the use of equation, whereas an increase in the MAD was seen in the other 2 groups, i.e., 11–30 and 51–70 years.
The sample was divided into 6 groups (10-year interval), namely, 11–20, 21–30, 31–40, 41–50, 51–60, and 61–70 years. Multilinear regression equations were obtained for samples belonging to each of these groups (Table 2).
By using the step-down analysis, it was possible to reduce the MAD from 7.945 obtained from equation 1 to an average MAD to 6. 33 obtained from the 10-band equation.
Following the calculation of MAD, the accuracy of the age estimated by the step-down analysis was evaluated. The age was estimated to within 5 years in 57/103 (55.33%), 5.1 to 10 years in 14/103 (13.39%), 10.1 to 15 years in 12/103 (11.65%), 15.1 to 20 years in 10/103 (9.7%), and more than 20 years in 10/103 (9.7%).
Recent studies along the similar lines have been made by Gupta et al. [20], Shah et al. [21], and Parra et al. [22]. Our study corroborates with that of Gupta et al. [20] which states that translucency of the root dentin can be used as a consistent factor for the age estimation. A recent study done by Shah et al. [21] also states that dentin translucency in the apical portion of the tooth can be used for assessing the age of a person. Parra et al. [22] state that the deteriorating changes in the root dentin translucency is not reliant on upon familial filiation or gender in any given age category. In our study, though genders of the patients were noted while taking the samples, gender-specific statistical analysis was not done as it was beyond the scope of this age-related study. Further analysis with focus on the gender can be done in future.
Post-hoc analysis for power calculation
This analysis revealed the requisite increase in number of samples in each of the age group in order to achieve a power calculation of 80% as only 57 samples out of 103 were correctly estimated with an accuracy of less than ±5 years.
The limitation of this study includes the difficulty in analyzing the 3D models of teeth that do not require any tooth sectioning and smaller sample size. Future studies with new software and a greater sample size can address this limitation.
In conclusion, the uniqueness of this study is that by making use of digital technology, the root dentinal translucency in “pixels” was measured, following which multilinear regression equations for specific age groups based on 10-year intervals were derived. By following a step-down analysis method, it was possible to estimate the age of an unknown sample with an average error of around ±7.9 years. Strategies for Age estimation in the near future would be the 3D manner of assessing root dentinal translucency without the need of sectioning the tooth and developing an algorithm for age estimation using software tools. This would give age estimation using digital technology a user-friendly approach.
XML Download