Introduction
Establishing a biological profile, which consists of sex, age, stature, and ethnicity, from unknown skeletal remains, is a crucial process in postmortem identification. This procedure assists in narrowing down the number of possible matches before applying a specific identification technique. Sex estimation is an important step in the identification of an unknown decedent because it influences other biological parameters, such as age and stature [
1]. Moreover, correct sex classification will reduce the number of possible missing persons by half [
1]. The sex of complete and well-preserved cadavers could simply be determined by external and internal genital organs. However, in the cases of severely decomposed, burned, dismembered and skeletonized human remains, bones are considered the second-best sex indicators.
The sex estimation methods for the skeletal remains are categorized into morphologic and metric. The sex determination from the pelvis is the most reliable morphologic method because it is proven to be the most sexually dimorphic skeletal element [
2]. No instrument is needed for the morphologic analysis although the accuracy significantly depends on the expertise of the observer [
3]. In addition, the incomplete condition of the bone will reduce the accuracy of the morphological method [
4]. Furthermore, a pelvis may not always be recovered or complete in some cases. In contrast, metric approaches for sexing skeletons yield low subjectivity, and the accuracy of the method relies less on the experience of the practitioner than that of morphological observation [
1,
3].
The measurements of long bone dimensions are actively studied for sex estimation using the metric techniques due to the sexual differences in size and robustness [
3]. Various long bones show the potential in estimating sex [
5]. Some studies reported that the dimensions of upper limb demonstrated higher ability to determine sex than those of lower limb [
6,
7]. In 2001, Mall et al. [
7] stated that radius performed the best among the three upper limb bones in sex estimation of samples from the contemporary German population. Similarly, previous studies also highlighted that the radius showed high degree of sexual dimorphism, and its dimensions could be used for estimating sex with high classification rates across many populations [
6-
13]. For instance, the antero-posterior diameter of the radial mid-shaft could predict the sex with the accuracy of 90.4% in the Indian population [
11]. The radial length demonstrated accuracies of 86.7% and 89.4% in discriminating sexes for the right and left sides in Greek population, respectively [
14].
Even though the radii may be promising candidates for metric sexing methods, most sex discriminant functions are derived from a specific population and may not be appropriate for different populations. Therefore, there is a need for developing a population-specific sex estimation function for each population. The assessment of sex using a complete radius in the northern Thai population was reported in 2004, with the accuracies of 86.9% and 89.4% for the right and left sides, respectively [
8]. However, as their methodology requires an intact radius to estimate the sex, it may not be applicable to forensic settings where radius might be recovered in a fragmented condition due to the decomposition process and scavenging. Therefore, the aim of this study was to establish the population-specific sex estimation method from the fragments of the radius for the Northern Thai population.
Go to :

Materials and Methods
Two hundred left-right pairs of radii (100 males and 100 females) were collected from the blind peer review. The source of radius bones were donated cadavers obtained from the northern region of Thailand. Exclusion criteria included bones with fractures, gross pathologic changes, and severe degenerative diseases. Sex, age at death, stature, race, occupation, and cause of death of all samples in this study were documented. The studied population consisted of individuals who were born between 1921 and 1995, and died between 2003 and 2015. The mean age for male samples was 63.92 years (range, 19–90 years); the mean age for female samples was 63.37 years old (range, 29–91 years). For age distribution of the samples used in this study, see
Table 1.
Table 1
The age distribution of the samples regarding to sex
|
Age range (yr) |
Male (n=100) |
|
Female (n=100) |
|
Total (n=200) |
|
Frequency |
Age (yr) |
Frequency |
Age (yr) |
Frequency |
Age (yr) |
|
10–19 |
0 |
- |
|
1 |
19 |
|
1 |
19 |
|
20–29 |
2 |
27.5±2.12 |
|
1 |
22 |
|
3 |
25.7±3.51 |
|
30–39 |
5 |
36.8±1.09 |
|
6 |
33.7±3.72 |
|
11 |
35.1±3.17 |
|
40–49 |
9 |
46.3±2.00 |
|
13 |
45.9±2.69 |
|
22 |
46.1±2.38 |
|
50–59 |
22 |
54.3±2.78 |
|
14 |
54.4±3.02 |
|
36 |
54.4±2.84 |
|
60–69 |
25 |
64.5±3.20 |
|
26 |
65.1±3.16 |
|
51 |
65.0±3.18 |
|
70–79 |
19 |
74.7±3.25 |
|
26 |
74.7±2.86 |
|
45 |
74.7±2.63 |
|
80–89 |
17 |
83.4±3.46 |
|
12 |
83.5±3.20 |
|
29 |
83.4±3.12 |
|
90–99 |
1 |
91.0 |
|
1 |
90.0 |
|
2 |
90.5±0.70 |

Additional 40 left-right pairs of radii (20 males and 20 females) were selected to evaluate the accuracy of sex discriminant functions. The mean age was 63.05 years (range, 36–78.2 years) for male test samples, and that of female test samples was 59.3 years (range, 43–76 years). The test samples for both sexes were born between 1924 and 1974, and died between 2005 and 2014. This study was approved by the Research Ethics Committee of the Faculty of Medicine, Chiang Mai University, Thailand (No. FOR-2560-04927). Study period in a research was one year for data collection and data analysis.
Measurement
Eight measurements were taken from the right and left radii. Four of the eight measurements were taken according to the standards presented by Suwanlikhid and Mahakanukrauh (2004) [
8] and Barrier and L’Abbé (2008) [
9], and the remaining four measurements were devised for this study. Each sample was measured by the forensic physician (WJ). Twenty pairs of training samples were randomly chosen and measured again by the forensic physician (WJ) and another forensic physician (MB) to evaluate the intra and inter-observer reliability. For the descriptions of the eight measurements used in this study, see
Table 2 and
Fig. 1.
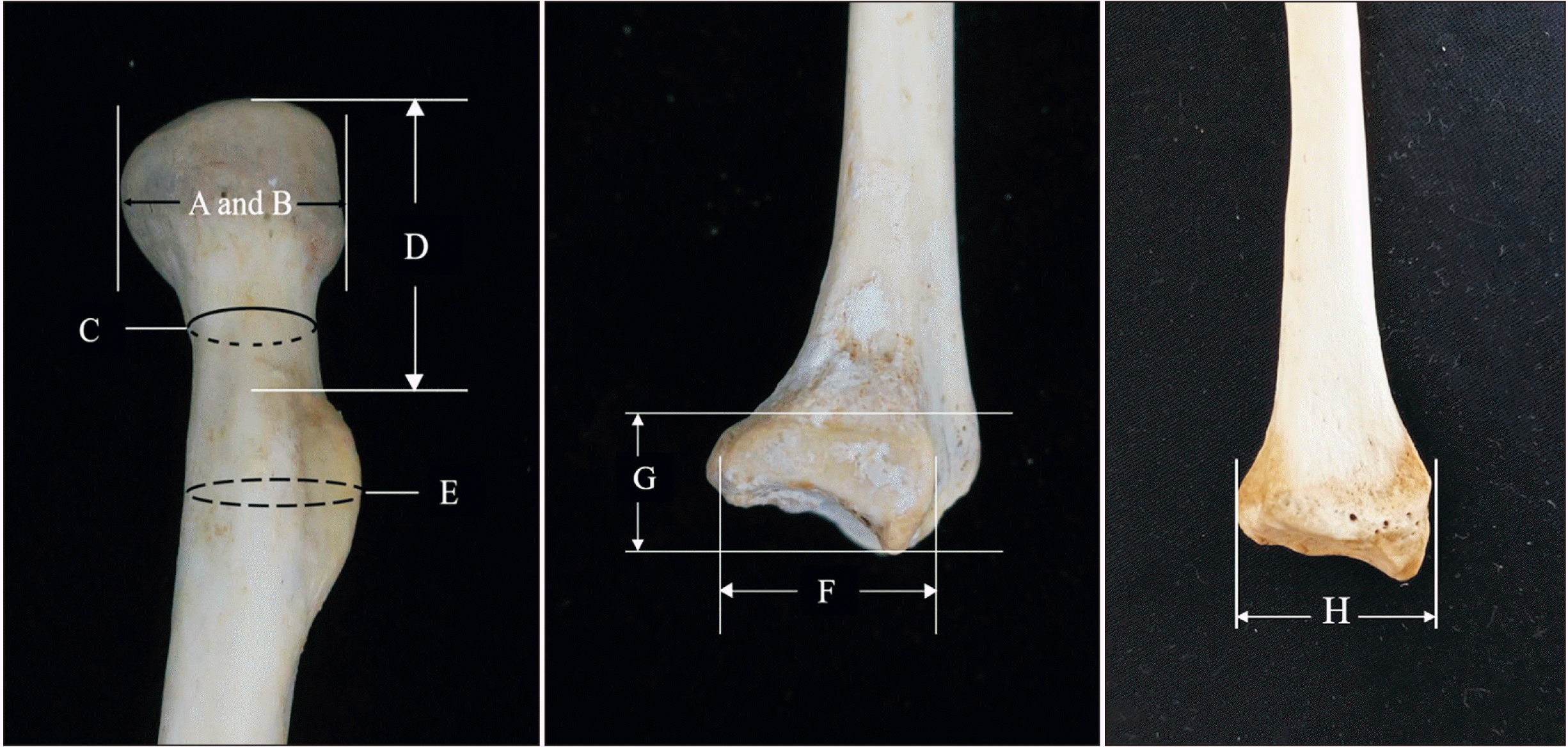 | Fig. 1Eight measurements of radius. A: maximum diameter of head, B: minimum diameter of head, C: circumference of neck, D: head-tuberosity length, E: circumference of tuberosity, F: ulnar notch length, G: ulnar notch width, H: distal end width of the radius. 
|
Table 2
The definition of the radial measurements
|
Measurement |
Description |
|
1. Maximum diameter of head |
Maximum value measured by digital Vernier caliper that rotates around the radial head [8, 9]. |
|
2. Minimum diameter of head |
Minimum value measured by digital Vernier caliper that rotates around the radial head [8, 9]. |
|
3. Circumference of neck |
Circumference at mid neck of radius (present study). The radius is placed with the anterior surface facing up. |
|
Circumference at mid radial neck were measured in millimeters by a standard tape. |
|
4. Head-tuberosity length |
Distance between the most proximal point on the head to the most proximal point on the tuberosity of the radius (present study). This measurement is taken by holding the radius so that the radial tuberosity faces towards the individual taking the measurement. The sliding calipers are positioned parallel to the longitudinal axis of the proximal radius with fixed arm on the most proximal point on the radial head. The calipers are then adjusted to meet the most proximal point on radial tuberosity. |
|
5. Circumference of tuberosity |
Circumference at mid tuberosity of radius [8]. |
|
6. Ulnar notch length |
Maximum distance between the most anterior and posterior side of ulnar notch (present study). |
|
7. Ulnar notch width |
Maximum distance between the most distal and proximal side of ulnar notch. (present study). |
|
8. Distal end width of the radius |
Maximum distance between the most medial and lateral point on the distal epiphysis of radius [8]. |

Statistics
The intra- and inter-observer errors were analyzed by the technical error of measurement (TEM), relative technical error of measurement (rTEM), and coefficient of reliability (R) [
15,
16]. The descriptive statistics, such as mean, standard deviation and range was used to describe the value of each measurement. Independent
t-test was performed to assess the differences in each measurement between both sexes, and normality of the data was tested by 1 sample Kolmogorov–Smirnov statistic. The measurements that showed statistically significant differences between both sexes were subjected to direct and stepwise discriminant function analyses. For the stepwise procedure, all selected variables were entered into a stepwise discriminant function using Wilks’ lambda, in order to determine which variables would provide the best discrimination between male and female groups. The discriminant functions were generated separately for the right and left sides. The accuracy of sex estimation functions was expressed in percentage. Statistical significance was observed at the
P-value below 0.05, and all data were analyzed by IBM SPSS Statistics ver. 22.0. (IBM Corp., Armonk, NY, USA).
Go to :

Results
The difference between observations is described in
Table 3. The R-value of all measurements was more than 0.9., and the ulnar notch width (UNW) demonstrated the highest percentage of rTEM for the inter-observer error whereas the highest percentage TEM for the intra-observer error was the ulnar notch length (UNL). However, none of the variables in which the value of rTEM was more than 1.5 for intra-observation error or more than 2 for inter observation error, which fell within the acceptable range [
16]. These results indicated that the devised measurements in this study were reliable and reproducible. The high values of rTEM in inter-observation error of UNW and the intra-observation error of UNL might be contributed to the unclear margin of ulnar notch.
Table 3
The technical error of measurement (TEM) and coefficient of reliability of intra and inter-observer error
|
Measurement |
Number |
Side |
Intra-observer error |
|
Inter-observer error |
|
TEM (mm) |
Relative TEM (%) |
R |
TEM (mm) |
Relative TEM (%) |
R |
|
Maximum diameter of head |
20 |
Left
Right |
0.06
0.07 |
0.29
0.34 |
0.999
0.999 |
|
0.11
0.15 |
0.51
0.72 |
0.997
0.994 |
|
Minimum diameter of head |
20 |
Left
Right |
0.08
0.08 |
0.43
0.42 |
0.998
0.998 |
|
0.08
0.09 |
0.43
0.46 |
0.998
0.998 |
|
Circumference of neck |
20 |
Left
Right |
0.36
0.35 |
0.90
0.88 |
0.993
0.994 |
|
0.27
0.35 |
0.67
0.86 |
0.996
0.995 |
|
Head-tuberosity length |
20 |
Left
Right |
0.18
0.18 |
0.86
0.86 |
0.994
0.994 |
|
0.29
0.30 |
1.31
1.38 |
0.980
0.981 |
|
Circumference of tuberosity |
20 |
Left
Right |
0.32
0.38 |
0.68
0.82 |
0.995
0.993 |
|
0.32
0.39 |
0.69
0.85 |
0.992
0.989 |
|
Ulnar notch width |
20 |
Left
Right |
0.12
0.10 |
1.02
0.86 |
0.996
0.996 |
|
0.22
0.23 |
1.83
1.95 |
0.989
0.979 |
|
Ulnar notch length |
20 |
Left
Right |
0.17
0.18 |
1.01
1.11 |
0.987
0.987 |
|
0.21
0.27 |
1.33
1.68 |
0.959
0.958 |
|
Distal end width of the radius |
20 |
Left
Right |
0.23
0.22 |
0.77
0.71 |
0.993
0.994 |
|
0.28
0.25 |
0.93
0.82 |
0.987
0.991 |

The dimensions of the right radius were larger than those of the left side in most parameters except head-tuberosity length (HTL) of female and circumference of the radial neck (CN), where the right side was smaller than the left side. Statistical significant differences between both sides were found at most dimorphic single parameter was maximum head diameter (MDH), minimum diameter of head (MNH), UNL and the distal end width of the radius (RDEW) in males, and CN and RDEW in females (
Table 4). To investigate the effect of outliers causing false statistical significance in the present data, boxplot diagram with median values, outliers, and standard deviation for MDH, MNH, UNL, RDEW in males and CN, RDEW in females were created (
Fig. 2). Outliers in the measurement data were identified, but the statistical significant difference between both sides was still observed even though the outliers were excluded from the analysis.
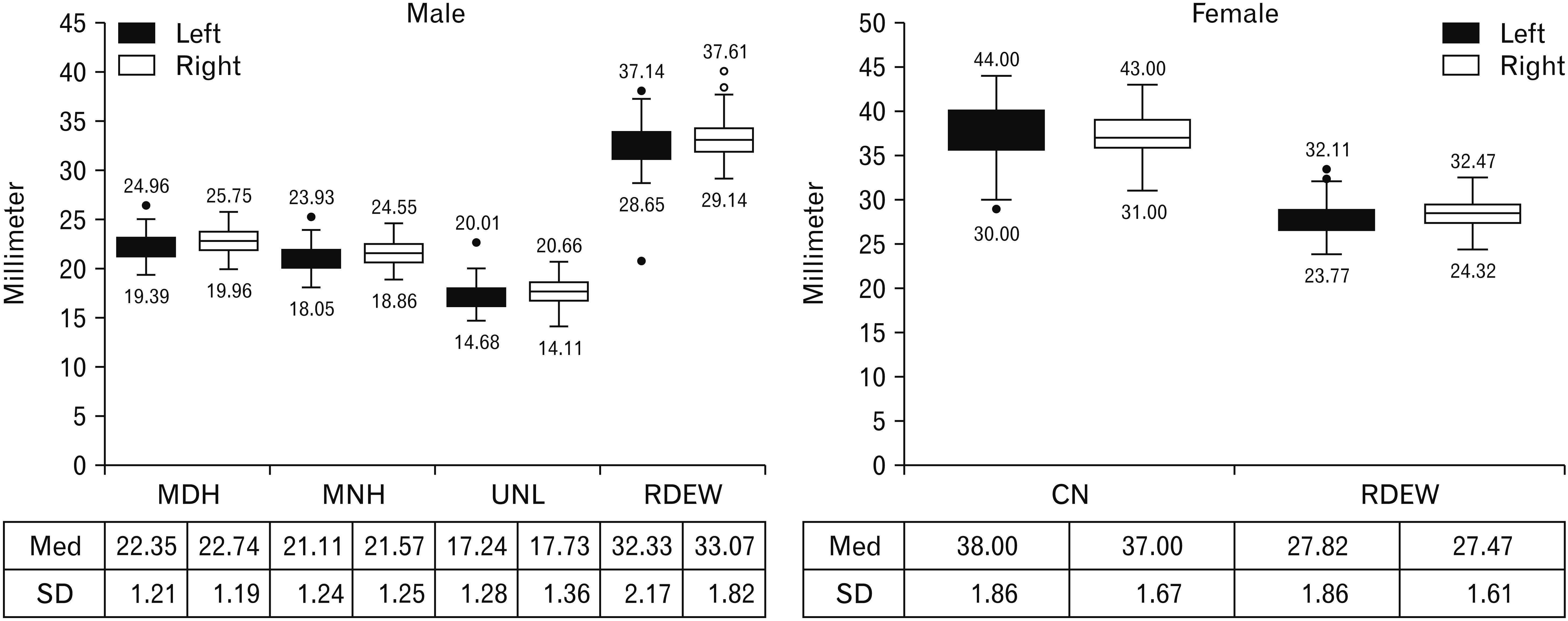 | Fig. 2The boxplot diagram shows median values (Med), outliers, and standard deviation (SD) of maximum diameter of head (MDH), minimum diameter of head (MNH), ulnar notch length (UNL), distal end width of the radius (RDEW) in male samples and circumference of neck (CN) and RDEW in female samples. 
|
Table 4
The average value of measurements according to the side and sex
|
Measurement (mm) |
Male |
|
Female |
|
Left |
Right |
P-value |
Left |
Right |
P-value |
|
Maximum diameter of head |
22.29 |
22.71 |
<0.001 |
|
19.36 |
19.43 |
0.396 |
|
Minimum diameter of head |
21.07 |
21.55 |
<0.001 |
|
18.35 |
18.42 |
0.385 |
|
Circumference of neck |
43.78 |
43.56 |
0.226 |
|
37.61 |
36.99 |
<0.001 |
|
Head-tuberosity length |
22.71 |
22.72 |
0.912 |
|
20.06 |
19.79 |
0.011 |
|
Circumference of tuberosity |
49.86 |
50.12 |
0.160 |
|
43.63 |
43.75 |
0.449 |
|
Ulnar notch width |
12.32 |
12.49 |
0.164 |
|
10.48 |
10.71 |
0.030 |
|
Ulnar notch length |
17.26 |
17.71 |
<0.001 |
|
15.54 |
15.73 |
0.039 |
|
Distal end width of the radius |
32.40 |
33.09 |
<0.001 |
|
27.86 |
28.41 |
<0.001 |

All measurements of males were significantly larger than those of females (
Fig. 3,
Table 5). The dimensions of the right radius demonstrated a higher t-value than those of the left side except for circumference of tuberosity (CT) (
Table 5). The MDH showed the highest sexual dimorphism (
t: 20.360) in right radius followed by RDEW (
t: 19.324) and MNH (
t: 19.278) while RDEW was the most sexually dimorphic parameter in the left radius (
t: 17.367) followed by MDH (
t: 17.192) and MNH (
t: 16.171). On the contrary, UNW indicated the lowest sexual dimorphism for both sides (
t: 9.129, 8.620). The result of 1 sample Kolmogorov–Smirnov test suggested all variables were distributed normally.
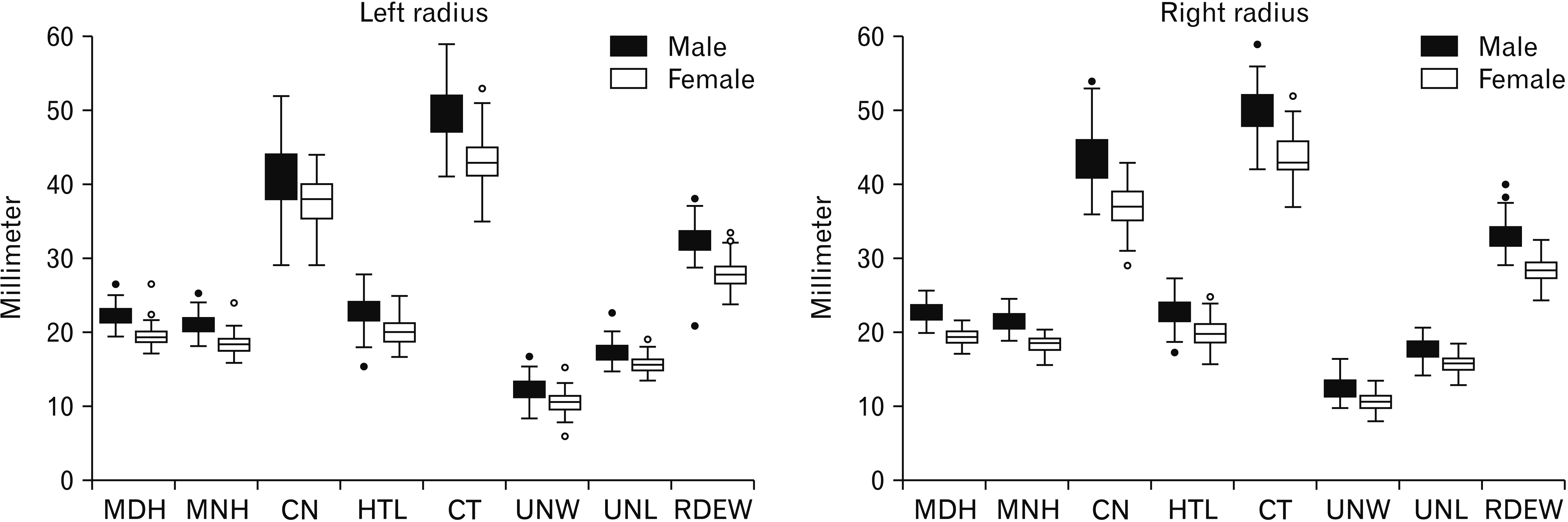 | Fig. 3The distribution of eight measurements of male and female samples for each side. MDH, maximum diameter of head; MNH, minimum diameter of head; CN, circumference of neck; HTL, head-tuberosity length; CT, circumference of tuberosity; UNW, ulnar notch width; UNL, ulnar notch length; RDEW, distal end width of the radius. 
|
Table 5
Descriptive statistics independent t-test differences between sexes of the variables
|
Measurement (mm) |
Side |
Male |
|
Female |
|
Independent t-test |
|
Min. |
Max. |
Mean |
SD |
Min. |
Max. |
Mean. |
SD |
t-value |
P
|
|
Maximum diameter of head |
Left |
19.39 |
26.52 |
22.29 |
1.27 |
17.03 |
24.49 |
19.36 |
1.14 |
17.192 |
<0.001 |
|
Right |
19.96 |
25.75 |
22.71 |
1.19 |
17.09 |
21.63 |
19.43 |
1.09 |
20.360 |
<0.001 |
|
Minimum diameter of head |
Left |
18.05 |
25.21 |
21.07 |
1.25 |
15.88 |
23.91 |
18.35 |
1.13 |
16.171 |
<0.001 |
|
Right |
18.86 |
24.55 |
21.55 |
1.26 |
15.66 |
20.42 |
18.42 |
1.03 |
19.278 |
<0.001 |
|
Circumference of neck |
Left |
36.00 |
52.00 |
43.78 |
3.36 |
29.00 |
44.00 |
37.61 |
2.91 |
13.878 |
<0.001 |
|
Right |
36.00 |
54.00 |
43.56 |
3.66 |
29.00 |
43.00 |
36.99 |
2.90 |
14.073 |
<0.001 |
|
Head-tuberosity length |
Left |
15.28 |
27.76 |
22.71 |
2.07 |
16.56 |
24.84 |
20.06 |
1.72 |
9.831 |
<0.001 |
|
Right |
17.32 |
27.37 |
22.72 |
1.89 |
15.61 |
24.82 |
19.79 |
1.74 |
11.404 |
<0.001 |
|
Circumference of tuberosity |
Left |
41.00 |
59.00 |
49.86 |
3.36 |
35.00 |
53.00 |
43.63 |
3.09 |
13.660 |
<0.001 |
|
Right |
42.00 |
61.00 |
50.12 |
3.48 |
37.00 |
52.00 |
43.75 |
3.16 |
13.546 |
<0.001 |
|
Ulnar notch width |
Left |
8.26 |
16.71 |
12.32 |
1.62 |
6.89 |
15.18 |
10.48 |
1.39 |
8.620 |
<0.001 |
|
Right |
9.79 |
16.43 |
12.49 |
1.56 |
8.01 |
13.45 |
10.71 |
1.18 |
9.129 |
<0.001 |
|
Ulnar notch length |
Left |
14.68 |
22.55 |
17.26 |
1.28 |
13.42 |
18.97 |
15.54 |
1.09 |
10.219 |
<0.001 |
|
Right |
14.11 |
20.66 |
17.71 |
1.36 |
12.89 |
18.58 |
15.73 |
1.16 |
11.079 |
<0.001 |
|
Distal end width of the radius |
Left |
20.80 |
38.07 |
32.40 |
1.28 |
23.77 |
33.39 |
27.86 |
1.86 |
17.367 |
<0.001 |
|
Right |
29.14 |
39.99 |
33.09 |
1.80 |
24.32 |
32.47 |
28.41 |
1.62 |
19.324 |
<0.001 |

The accuracy rates of sex estimation functions using single parameter of the radius in this study ranged from 73.0% to 92.0%, and most of the radial dimensions could predict the sex with accuracy over 80% except for both sides of the UNW, UNL and left HTL (
Table 6). Some of the devised measurements in this study, namely CN and HTL, indicated high ability to predict sex. The CN performed the best among the four devised radial measurements in correct sex classification, in which the percentage of accuracy of right and left were 86.5% and 85.5%, respectively. The sex discriminant function for right HTL provided the accuracy of 81.5% whereas left HTL could predict the sex with the accuracy of only 77.0%. The UNW showed the lowest ability to predict the sex among the devised and all measurements in this study, in which the classification rates were 73.0% and 74.0% for the right and left sides, respectively. The MDH displayed the highest ability to estimate sex in this study, with accuracies of 92.0% and 90.5% for the right and left sides, respectively.
Table 6
Direct discriminant function percent of accuracy and corrected percentage of accuracy for radius
|
Function |
Variable |
Unstandardized
coefficients |
Group centroid |
Predicted group |
Accuracy (%) |
|
M |
F |
Total |
|
Right |
|
|
|
|
|
|
|
|
1 |
MDH
Constant |
0.876
–18.465 |
M=1.440
F=–1.440 |
Original
Cross-validated |
92.0
92.0 |
92.0
92.0 |
92.0
92.0 |
|
2 |
MNH
Constant |
0.871
–17.417 |
M=1.363
F=–1.363 |
Original
Cross-validated |
90.0
90.0 |
93.0
93.0 |
91.5
91.5 |
|
3 |
CN
Constant |
0.303
–12.201 |
M=0.995
F =–0.995 |
Original
Cross-validated |
81.0
81.0 |
92.0
92.0 |
86.5
86.5 |
|
4 |
HTL
Constant |
0.550
–11.698 |
M=0.806
F=–0.806 |
Original
Cross-validated |
83.0
83.0 |
80.0
80.0 |
81.5
81.5 |
|
5 |
CT
Constant |
0.310
–14.115 |
M=0.958
F=–0.958 |
Original
Cross-validated |
86.0
86.0 |
82.0
82.0 |
84.0
84.0 |
|
6 |
UNW
Constant |
0.724
–8.397 |
M=0.646
F=–0.646 |
Original
Cross-validated |
69.0
69.0 |
77.0
77.0 |
73.0
73.0 |
|
7 |
UNL
Constant |
0.792
–13.244 |
M=0.783
F=–0.783 |
Original
Cross-validated |
75.0
75.0 |
83.0
83.0 |
79.0
79.0 |
|
8 |
RDEW
Constant |
0.584
–17.953 |
M=1.366
F=–1.366 |
Original
Cross-validated |
91.0
91.0 |
93.0
92.0 |
92.0
91.5 |
|
Left |
|
|
|
|
|
|
|
|
9 |
MDH
Constant |
0.831
–17.295 |
M=1.216
F=–1.216 |
Original
Cross-validated |
90.0
90.0 |
91.0
91.0 |
90.5
90.5 |
|
10 |
MNH
Constant |
0.840
–16.568 |
M=1.143
F=–1.143 |
Original
Cross-validated |
87.0
87.0 |
94.0
94.0 |
90.5
90.5 |
|
11 |
CN
Constant |
0.318
–12.944 |
M=0.981
F=–0.981 |
Original
Cross-validated |
86.0
86.0 |
85.0
85.0 |
85.5
85.5 |
|
12 |
HTL
Constant |
0.525
–11.237 |
M=0.695
F=–0.695 |
Original
Cross-validated |
77.0
77.0 |
77.0
77.0 |
77.0
77.0 |
|
13 |
CT
Constant |
0.310
–14.494 |
M=0.966
F=–0.966 |
Original
Cross-validated |
82.0
82.0 |
82.0
82.0 |
82.0
82.0 |
|
14 |
UNW
Constant |
0.663
–7.559 |
M=0.609
F=–0.609 |
Original
Cross-validated |
72.0
72.0 |
77.0
76.0 |
74.5
74.0 |
|
15 |
UNL
Constant |
0.843
–13.827 |
M=0.723
F=–0.723 |
Original
Cross-validated |
72.0
72.0 |
79.0
79.0 |
75.5
75.5 |
|
16 |
RDEW
Constant |
0.540
–16.283 |
M=1.228
F=–1.228 |
Original
Cross-validated |
89.0
89.0 |
90.0
89.0 |
89.5
89.0 |

Stepwise discriminant function analysis was carried out for all measurements and for different segments of radius (proximal and distal). The right radius showed higher ability to estimate sex than the left, and the accuracy rate of proximal part was higher than that of distal part of radius. MDH, CN, HTL, and RDEW were chosen for stepwise discriminant functions for both sides as these parameters presented high classification accuracies of 96.0% and 95.0% for the right and left sides, respectively. The proximal radius could be used for sex estimation with accuracy rates of 95.0% for the right radius and 93.0% for the left radius. MDH, CN and HTL were selected for sex estimation function for the right proximal radius whereas the parameters of the function for left proximal radius were MDH, HTL and CT. The sex estimation functions of distal radius provided accuracy rates of 92.5% and 89.5% for the right and left radius, respectively; UNW, UNL and RDEW were selected for right distal radius while only UNW and RDEW were chosen for left distal radius (
Table 7).
Table 7
Stepwise discriminant function, percent of accuracy and corrected percentage of accuracy for radius
|
Function |
Variable |
Unstandardized
coefficient |
Group centroid |
Predicted group |
Accuracy (%) |
|
M |
F |
Total |
|
17 |
Right radius |
MDH
CN
HTL
RDEW
Constant |
0.332
0.090
0.204
0.286
–23.754 |
M=1.810
F=–1.810 |
Original
Cross-validated |
96.0
96.0 |
96.0
96.0 |
96.0
96.0 |
|
18 |
Right radius |
MDH
CN
HTL
Constant |
0.569
0.101
0.243
–21.216 |
M=1.622
F=–1.622 |
Original
Cross-validated |
95.0
95.0 |
95.0
95.0 |
95.0
95.0 |
|
19 |
Right distal |
UNW
UNL
RDEW
Constant |
0.144
0.181
0.485
–19.611 |
M=1.443
F=–1.443 |
Original
Cross-validated |
91.0
91.0 |
94.0
94.0 |
92.5
92.5 |
|
20 |
Left radius |
MDH
CN
HTL
RDEW
Constant |
0.270
0.111
0.205
0.271
–22.667 |
M=1.623
F=–1.623 |
Original
Cross-validated |
95.0
95.0 |
96.0
95.0 |
95.5
95.0 |
|
21 |
Left proximal |
MDH
HTL
CT
Constant |
0.524
0.210
0.137
–21.810 |
M=1.472
F=–1.472 |
Original
Cross-validated |
94.0
94.0 |
92.0
92.0 |
93.0
93.0 |
|
22 |
Left distal |
UNW
RDEW
Constant |
0.167
0.490
–16.658 |
M=1.266
F=–1.266 |
Original
Cross-validated |
90.0
90.0 |
89.0
89.0 |
89.5
89.5 |

The sectioning point of the discriminant functions was calculated from the average of the summation of group centroid value. In the present study, the sectioning point of all functions was 0, so if the score of the discriminant function analysis was more than 0, then the sample would be classified as male whereas if the score was less than 0, the result would suggest female. On the test sample, the accuracy of the single-variable sex discriminant function for the right radius ranged from 85.0% to 97.5% and around 85.0% to 95.0% for the left radius, which performed better than those of the studied samples (
Table 8). MDH was still regarded the best sex discriminator for the right radius although MNH, CT, and UNL indicated the highest classification accuracy for the left radius among the test samples. Multivariate discriminant function increased the accuracy rate of sex prediction for both sides, and the accuracy rates of sex estimation function for the right radius and proximal part were better than those of the left radius and distal part, as illustrated by the results of the training samples.
Table 8
The accuracy of testing sex discriminant function to the test samples
|
Function |
Variable |
Accuracy (%) |
|
Male |
Female |
Total |
|
Right |
|
|
|
|
|
1 |
MDH |
95.0 |
100.0 |
97.5 |
|
2 |
MNH |
95.0 |
95.0 |
95.0 |
|
3 |
CN |
90.0 |
90.0 |
90.0 |
|
4 |
HTL |
85.0 |
85.0 |
85.0 |
|
5 |
CT |
90.0 |
90.0 |
90.0 |
|
6 |
UNW |
87.5 |
87.5 |
87.5 |
|
7 |
UNL |
90.0 |
90.0 |
90.0 |
|
8 |
RDEW |
95.0 |
95.0 |
95.0 |
|
17 |
MDH, CN, HTL, RDEW |
95.0 |
100.0 |
97.5 |
|
18 |
MDH, CN, HTL |
100.0 |
100.0 |
100.0 |
|
19 |
UNW, UNL, RDEW |
90.0 |
95.0 |
92.5 |
|
Left |
|
|
|
|
|
9 |
MDH |
90.0 |
90.0 |
90.0 |
|
10 |
MNH |
95.0 |
95.0 |
95.0 |
|
11 |
CN |
92.5 |
92.5 |
92.5 |
|
12 |
HTL |
85.0 |
85.0 |
85.0 |
|
13 |
CT |
95.0 |
95.0 |
95.0 |
|
14 |
UNW |
90.0 |
90.0 |
90.0 |
|
15 |
UNL |
95.0 |
95.0 |
95.0 |
|
16 |
RDEW |
85.0 |
85.0 |
85.0 |
|
20 |
MDH, CN, HTL, RDEW |
90.0 |
100.0 |
95.0 |
|
21 |
MDH, HTL, CT |
95.0 |
100.0 |
97.5 |
|
22 |
UNL, RDEW |
85.0 |
100.0 |
92.5 |

Go to :

Discussion
Identification of the skeletal fragments remains a challenging task for forensic pathologists. According to our results, fragmented radius showed potential for sex estimation with a high classification rate, and the devised measurements of this study were reproducible without significant errors. The right radius indicated higher ability to predict the sex than the left side while proximal part was more reliable than the distal part for sex determination in fragmented radius.
The high sex discrimination rate in the present study is consistent with previous studies using radius for sex estimation in various populations (
Table 9) [
6,
7,
10,
12,
14,
17,
18]. In a study of German population, Mall et al. [
7] found that maximum diameter of head, maximum length, and distal end width of the radius produced a high accuracy of 94.93% when all three variables were applied together. Moreover, the correct sex discriminant rates for Japanese [
17], Greek [
14], Turkish [
10], and British medieval populations [
12] were also over 90%. However, the level of accuracy was only 86.5% for complete radius from South African samples whereas the incomplete radius resulted in 86% of accuracy [
9]. In addition, the accuracy of sex estimation function using radius from Indian and archaeological Polish population was less than 90% [
9,
11,
13]. The discrepancy might be caused by the variation across different populations, in which differential environmental and genetic factors affect the degree of sexual dimorphism expression in each population [
19]. Moreover, inconsistent standard measurements in various studies may also affect the accuracy of sex estimation model.
Table 9
Comparison the percentage of accuracy of sex estimation using radial dimension with other populations
|
Author (yr) |
Population |
Parameter |
Accuracy (%) |
|
Mall et al. (2001) [7] |
German |
MDH, MRL, RDEW |
88.6–89.1 |
|
Safont et al. (2000) [6] |
Late Roman period site of Mas Rimbau/Mas Mallol |
CT, Msc |
90.0–93.0 |
|
Suwanlikhid and Mahakkanukrauh (2004) [8] |
Northern Thai |
MRL, MDH, MNH, RDEW, W, HC, CT, Msc |
86.9–89.4 |
|
Sakaue (2004) [17] |
Japanese |
MRL, SHD, THD, RDEW, NB, MSA |
80.0–98.0 |
|
Barrier and L’Abbé (2008) [9] |
South African |
MRL, RDEW, Min-Ms, Max-Ms, VHD, MDH, MNH, HC, CT |
81.0–86.5 |
|
Charisi et al. (2011) [14] |
Greek |
MRL, MRPW, RDEW |
93.5–95.1 |
|
Uzün et al. (2011) [10] |
Turkish |
MRL, Ms-AP, Ms-tran, RDEW |
90.4–91.9 |
|
Waghmare et al. (2012) [11] |
Indian |
MSc, Ms-AP, Ms-tran, CT, AP-RT, V-RT |
71.7–90.4 |
|
Martin et al. (2016) [12] |
British medieval populations |
MRL, MRPW, RDEW |
91.2–95.5 |
|
Duangto and Mahakkanukrauh (2020) [18] |
Northern Thai |
MRL, Ms-AP, MLR |
77.6–95.2 |
|
Current study (2020) |
Northern Thai |
MDH, MNH, CN, CT, UNL, UNW, RDEW |
76.0–96.0 |

Most of the radial dimensions in this study, such as MDH, CT, HTL and RDEW, were smaller than those of other populations [
6,
7,
9,
10,
14]. However, our results suggested that the dimensions of radius still demonstrated significant sexual dimorphism in the Northern Thai population. MDH indicated the highest sexual dimorphism of all measurements used in this study, in which the classification rate of right MDH was 92% and 90.5% for the left side. The accuracy rate was higher than those of German and South African populations [
7,
9]. The sex classification accuracy of MNH was equal to left MDH (90.5%), and the classification rate of MNH was equal to right RDEW (91.5%). However, there was no report of accuracy rate for single variable sex estimation function using MNH to compare with other populations. CT showed fair accuracy in estimating sex in Thai population, but the classification rate was dramatically lower than those of archaeological Spanish population (92.8%) [
6]. The RDEW was the best discriminant parameter for distal radius, in which the accuracy rates were 91.5% and 89% for the right and left sides, respectively. The accuracy of sex estimation using RDEW in this study was higher than those of German and South African populations but slightly lower than those of European white and black samples [
7,
9,
20].
The average dimensions of MDH, MNH, CT and RDEW, which were also used for estimating sex in previous study on the Northern Thai population [
8], in our study were different from the aforementioned report. However, the significant difference was only found in the RDEW and MNH dimensions. The dimensions of right and left RDEW in male samples in our study were 2.72 mm and 3.02 mm larger than those of previous study, respectively (
t-test,
P<0.001). For female samples, the dimensions of right and left RDEW were 1.79 mm and 1.88 mm larger than those of previous study, respectively (
t-test,
P<0.001). The average of left MNH from male samples was 0.51 mm larger than those of previous study (
t-test,
P<0.05) but the average of right MNH from female samples was 0.37 mm smaller than those of previous study (
t-test,
P<0.05). The different in size of present and past study might be explained by the different of sample selection and the secular changes in Thai population [
21]. Our report studied the individuals who died between 2003 and 2015 whereas the previous report obtained the specimens who died between 1993 and 2001. In addition, the difference in overall living conditions between these two generational populations might impact skeletal dimensions [
21].
Bilateral asymmetry was detected in some dimensions among our samples; the right radius was more accurate in predicting sex than left radius. The result of this study is consistent with the previous study focused on Greek population [
14], in which maximum radial length, maximum radial proximal width, and maximum radial distal width of right radius could estimate sex with higher accuracy than those of left side. However, the sex estimation functions using maximum head diameter and midshaft circumference of left radius were more accurate than those of right radius in the Northern Thai population [
8].
The accuracy of sex estimation functions using proximal radius was higher than those of distal radius in the present study. This phenomenon could be contributed to the fact that the proximal part was more affected by a carrying angle of the elbow, where sexual dimorphism is significantly expressed, than the distal part of radius [
17].
The sex discriminant functions in our study are applicable to both complete and incomplete radius of modern Thai skeletons, particularly, from the Northern Thailand region. Nevertheless, the validity of sex estimation functions of tarsal bone, derived from the Northern Thai population, was examined on the Northeastern Thai population in another study [
22]. The results suggested that the sex estimation functions derived from Northern Thai samples could also predict the sex in the Northeastern Thai population with high accuracy. However, the misclassification usually occurred among the Northeastern Thai samples that had greater height than the maximum height of the Northern Thai samples [
22]. Therefore, the application of sex discriminant functions from this study can potentially extend to other Thai regional populations although caution must be taken when the estimated height is higher than the maximum height in this study (190 cm for male and 170 cm for female).
The use of radial fragments in sex estimation was investigated on the Northern Thai population. This study provides sex estimation functions for various measurements that can be applied to radius from Northern Thai individuals with accuracy over 85%. The stepwise discriminant function analysis of proximal and distal radius could improve the accuracy of sex estimation functions to more than 90%.
Go to :








 PDF
PDF Citation
Citation Print
Print



 XML Download
XML Download