1. World Health Organization. Coronavirus Disease 2019 (COVID-19): Situation Report, 88. Geneva: World Health Organization;2020.
2. Maliszewska M, Mattoo A, Van Der Mensbrugghe D. The Potential Impact of COVID-19 on GDP and Trade: a Preliminary Assessment. World Bank Policy Research Working Paper. Washington, D.C.: The World Bank;2020.
3. Mizumoto K, Kagaya K, Zarebski A, Chowell G. Estimating the asymptomatic proportion of coronavirus disease 2019 (COVID-19) cases on board the Diamond Princess cruise ship, Yokohama, Japan, 2020. Euro Surveill. 2020; 25(10):2000180.

4. Day M. Covid-19: identifying and isolating asymptomatic people helped eliminate virus in Italian village. BMJ. 2020; 368:m1165. PMID:
32205334.

5. Ki M. Task Force for 2019-nCoV. Epidemiologic characteristics of early cases with 2019 novel coronavirus (2019-nCoV) disease in Korea. Epidemiol Health. 2020; 42:e2020007. PMID:
32035431.

6. Korean Society of Infectious Diseases. Korean Society of Pediatric Infectious Diseases. Korean Society of Epidemiology. Korean Society for Antimicrobial Therapy. Korean Society for Healthcare-associated Infection Control and Prevention. Korea Centers for Disease Control and Prevention. Report on the Epidemiological features of coronavirus disease 2019 (COVID-19) outbreak in the Republic of Korea from January 19 to March 2, 2020. J Korean Med Sci. 2020; 35(10):e112. PMID:
32174069.
8. Lauer SA, Grantz KH, Bi Q, Jones FK, Zheng Q, Meredith HR, et al. The incubation period of coronavirus disease 2019 (COVID-19) from publicly reported confirmed cases: estimation and application. Ann Intern Med. 2020; 172(9):577–582. PMID:
32150748.

9. Pan Y, Zhang D, Yang P, Poon LL, Wang Q. Viral load of SARS-CoV-2 in clinical samples. Lancet Infect Dis. 2020; 20(4):411–412. PMID:
32105638.

10. Gyeonggi Infectious Disease Control Center. Coronavirus Disease 2019 (COVID-19) Gyeonggi Daily Report, Data as Reported by 5 April 2020. Seongnam: Gyeonggi Infectious Disease Control Center;2020.
11. Morton A, Finkenstädt BF. Discrete time modelling of disease incidence time series by using Markov chain Monte Carlo methods. J R Stat Soc C Appl. 2005; 54(3):575–594.

12. O'Neill PD. A tutorial introduction to Bayesian inference for stochastic epidemic models using Markov chain Monte Carlo methods. Math Biosci. 2002; 180(1-2):103–114. PMID:
12387918.
13. Hellewell J, Abbott S, Gimma A, Bosse NI, Jarvis CI, Russell TW, et al. Feasibility of controlling COVID-19 outbreaks by isolation of cases and contacts. Lancet Glob Health. 2020; 8(4):e488–96. PMID:
32119825.

14. Pastorino B, Touret F, Gilles M, de Lamballerie X, Charrel RN. Evaluation of heating and chemical protocols for inactivating SARS-CoV-2. bioRxiv. Forthcoming. 2020; DOI:
10.1101/2020.04.11.036855.

15. Ferguson N, Laydon D, Nedjati-Gilani G, Imai N, Ainslie K, Baguelin M, et al. Impact of Non-pharmaceutical Interventions (NPIs) to Reduce COVID-19 Mortality and Healthcare Demand. London: Imperial College COVID-19 Response Team;2020.
16. Kraemer MU, Yang CH, Gutierrez B, Wu CH, Klein B, Pigott DM, et al. The effect of human mobility and control measures on the COVID-19 epidemic in China. Science. 2020; 368(6490):493–497. PMID:
32213647.

17. Lai S, Ruktanonchai NW, Zhou L, Prosper O, Luo W, Floyd JR, et al. Effect of non-pharmaceutical interventions for containing the COVID-19 outbreak in China. medRxiv. Forthcoming. 2020; DOI:
10.1101/2020.03.03.20029843.

18. Kim S, Kim YJ, Peck KR, Jung E. School opening delay effect on transmission dynamics of coronavirus disease 2019 in Korea: based on mathematical modeling and simulation study. J Korean Med Sci. 2020; 35(13):e143. PMID:
32242349.

19. Prem K, Cook AR, Jit M. Projecting social contact matrices in 152 countries using contact surveys and demographic data. PLOS Comput Biol. 2017; 13(9):e1005697. PMID:
28898249.

20. Liu Y, Yan LM, Wan L, Xiang TX, Le A, Liu JM, et al. Viral dynamics in mild and severe cases of COVID-19. Lancet Infect Dis. 2020; 20(6):656–657. PMID:
32199493.

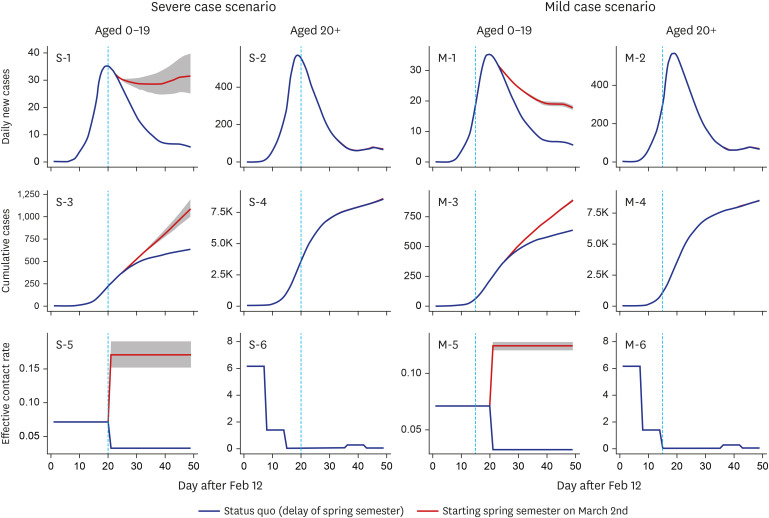
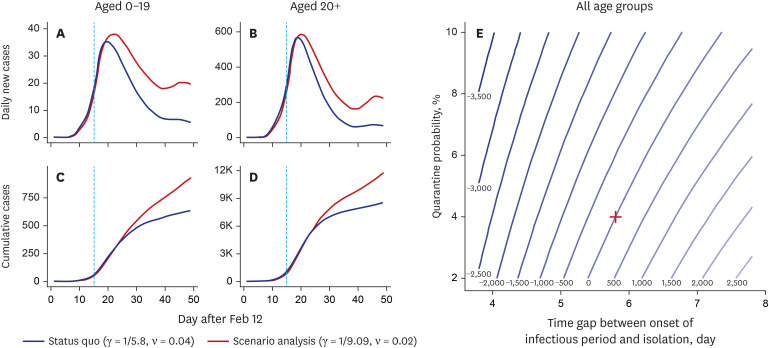
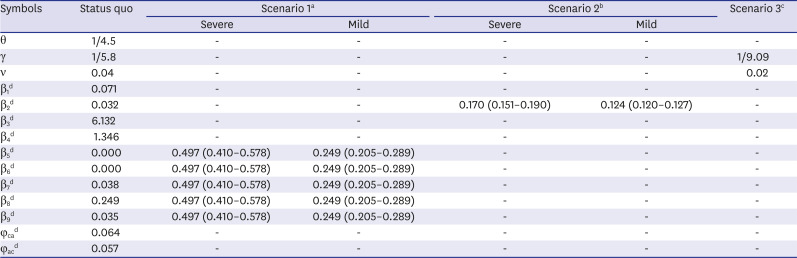
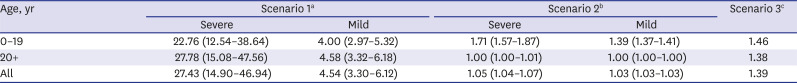





 PDF
PDF Citation
Citation Print
Print



 XML Download
XML Download