1. Joda T, Katsoulis J, Brägger U. Clinical fitting and adjustment time for implant-supported crowns comparing digital and conventional workflows. Clin Implant Dent Relat Res. 2016; 18:946–954. PMID:
26395013.

2. Baig MR. Accuracy of impressions of multiple implants in the edentulous arch: a systematic review. Int J Oral Maxillofac Implants. 2014; 29:869–880. PMID:
25032767.

3. Mormann WH, Brandestini M, Lutz F, Barbakow F, Gotsch T. CAD-CAM ceramic inlays and onlays: a case report after 3 years in place. J Am Dent Assoc. 1990; 120:517–520. PMID:
2186072.

4. Zarauz C, Valverde A, Martinez-Rus F, Hassan B, Pradies G. Clinical evaluation comparing the fit of all-ceramic crowns obtained from silicone and digital intraoral impressions. Clin Oral Investig. 2016; 20:799–806.

5. Rutkūnas V, Gečiauskaitė A, Jegelevičius D, Vaitiekūnas M. Accuracy of digital implant impressions with intraoral scanners. A systematic review. Eur J Oral Implantol. 2017; 10:101–120. PMID:
28944372.
6. Biagioni A, Ferrari M, Pecciarini M. A systematic review about randomized clinical trials on digital impressions on sound teeth. J Osseointegration. 2019; 11:2–6.
7. Flügge T, van der Meer WJ, Gonzalez BG, Vach K, Wismeijer D, Wang P. The accuracy of different dental impression techniques for implant-supported dental prostheses: A systematic review and meta-analysis. Clin Oral Implants Res. 2018; 29:374–392. PMID:
30328182.

8. Cappare P, Sannino G, Minoli M, Montemezzi P, Ferrini F. Conventional versus digital impressions for full arch screw-retained maxillary rehabilitations: A randomized clinical trial. Int J Environ Res Public Health. 2019; 16:E829. PMID:
30866465.

9. Alsharbaty MHM, Alikhasi M, Zarrati S, Shamshiri AR. A clinical comparative study of 3-dimensional accuracy between digital and conventional implant impression techniques. J Prosthodont. 2019; 28:e902–e908. PMID:
29423969.

10. Andriessen FS, Rijkens DR, van der Meer WJ, Wismeijer DW. Applicability and accuracy of an intraoral scanner for scanning multiple implants in edentulous mandibles: a pilot study. J Prosthet Dent. 2014; 111:186–194. PMID:
24210732.

11. Rutkunas V, Ignatovic J. A technique to splint and verify the accuracy of implant impression copings with light-polymerizing acrylic resin. J Prosthet Dent. 2014; 111:254–256. PMID:
24445030.

12. Abduo J, Bennani V, Waddell N, Lyons K, Swain M. Assessing the fit of implant fixed prostheses: a critical review. Int J Oral Maxillofac Implants. 2010; 25:506–515. PMID:
20556249.
13. Flügge TV, Att W, Metzger MC, Nelson K. Precision of dental implant digitization using intraoral scanners. Int J Prosthodont. 2016; 29:277–283. PMID:
27148990.

14. Gherlone EF, Ferrini F, Crespi R, Gastaldi G, Capparé P. Digital impressions for fabrication of definitive “all-on-four” restorations. Implant Dent. 2015; 24:125–129. PMID:
25621560.

15. Venezia P, Torsello F, Cavalcanti R, Casiello E, Chiapasco M. Digital registration of peri-implant transmucosal portion and pontic area in the esthetic zone. J Osseointegration. 2017; 9:312–316.
16. Abduo J. Fit of CAD/CAM implant frameworks: a comprehensive review. J Oral Implantol. 2014; 40:758–766. PMID:
25506666.

17. Beuer F, Schweiger J, Edelhoff D. Digital dentistry: an overview of recent developments for CAD/CAM generated restorations. Br Dent J. 2008; 204:505–511. PMID:
18469768.

18. Kohorst P, Brinkmann H, Li J, Borchers L, Stiesch M. Marginal accuracy of four-unit zirconia fixed dental prostheses fabricated using different computer-aided design/computer-aided manufacturing systems. Eur J Oral Sci. 2009; 117:319–325. PMID:
19583762.

19. Papaspyridakos P, Chen CJ, Gallucci GO, Doukoudakis A, Weber HP, Chronopoulos V. Accuracy of implant impressions for partially and completely edentulous patients: a systematic review. Int J Oral Maxillofac Implants. 2014; 29:836–845. PMID:
25032763.

20. Brånemark PI. Osseointegration and its experimental background. J Prosthet Dent. 1983; 50:399–410. PMID:
6352924.
21. Jemt T. Failures and complications in 391 consecutively inserted fixed prostheses supported by Brånemark implants in edentulous jaws: a study of treatment from the time of prosthesis placement to the first annual checkup. Int J Oral Maxillofac Implants. 1991; 6:270–276. PMID:
1813395.
22. Katsoulis J, Takeichi T, Sol Gaviria A, Peter L, Katsoulis K. Misfit of implant prostheses and its impact on clinical outcomes. Definition, assessment and a systematic review of the literature. Eur J Oral Implantol. 2017; 10:121–138. PMID:
28944373.
23. Karl M, Taylor TD. Bone adaptation induced by non-passively fitting implant superstructures: A randomized clinical trial. Int J Oral Maxillofac Implants. 2016; 31:369–375. PMID:
27004282.

24. Nedelcu R, Olsson P, Nyström I, Rydén J, Thor A. Accuracy and precision of 3 intraoral scanners and accuracy of conventional impressions: A novel in vivo analysis method. J Dent. 2018; 69:110–118. PMID:
29246490.

25. Semper W, Kraft S, Mehrhof J, Nelson K. Impact of abutment rotation and angulation on marginal fit: theoretical considerations. Int J Oral Maxillofac Implants. 2010; 25:752–758. PMID:
20657870.
26. Alikhasi M, Monzavi A, Bassir SH, Naini RB, Khosronedjad N, Keshavarz S. A comparison of precision of fit, rotational freedom, and torque loss with copy-milled zirconia and prefabricated titanium abutments. Int J Oral Maxillofac Implants. 2013; 28:996–1002. PMID:
23869357.

27. Flügge TV, Att W, Metzger MC, Nelson K. Precision of dental implant digitization using intraoral scanners. Int J Prosthodont. 2016; 29:277–283. PMID:
27148990.

28. Papaspyridakos P, Benic GI, Hogsett VL, White GS, Lal K, Gallucci GO. Accuracy of implant casts generated with splinted and non-splinted impression techniques for edentulous patients: an optical scanning study. Clin Oral Implants Res. 2012; 23:676–681. PMID:
21631595.

29. Tan MY, Yee SHX, Wong KM, Tan YH, Tan KBC. Comparison of three-dimensional accuracy of digital and conventional implant impressions: Effect of interimplant distance in an edentulous arch. Int J Oral Maxillofac Implants. 2019; 34:366–380. PMID:
30521661.

31. Giménez B, Özcan M, Martínez-Rus F, Pradíes G. Accuracy of a digital impression system based on active triangulation technology with blue light for implants: Effect of clinically relevant parameters. Implant Dent. 2015; 24:498–504. PMID:
26057777.
32. Gimenez-Gonzalez B, Hassan B, Özcan M, Pradíes G. An in vitro study of factors influencing the performance of digital intraoral impressions operating on active wavefront sampling technology with multiple implants in the edentulous maxilla. J Prosthodont. 2017; 26:650–655. PMID:
26934046.

33. Müller P, Ender A, Joda T, Katsoulis J. Impact of digital intraoral scan strategies on the impression accuracy using the TRIOS Pod scanner. Quintessence Int. 2016; 47:343–349. PMID:
26824085.
34. Stimmelmayr M, Güth JF, Erdelt K, Edelhoff D, Beuer F. Digital evaluation of the reproducibility of implant scanbody fit-an in vitro study. Clin Oral Investig. 2012; 16:851–856.

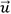 and
and 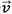 are direction vectors of the lines u and v.
are direction vectors of the lines u and v.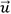 and
and 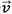 are direction vectors of the lines u and v.
are direction vectors of the lines u and v.