Abstract
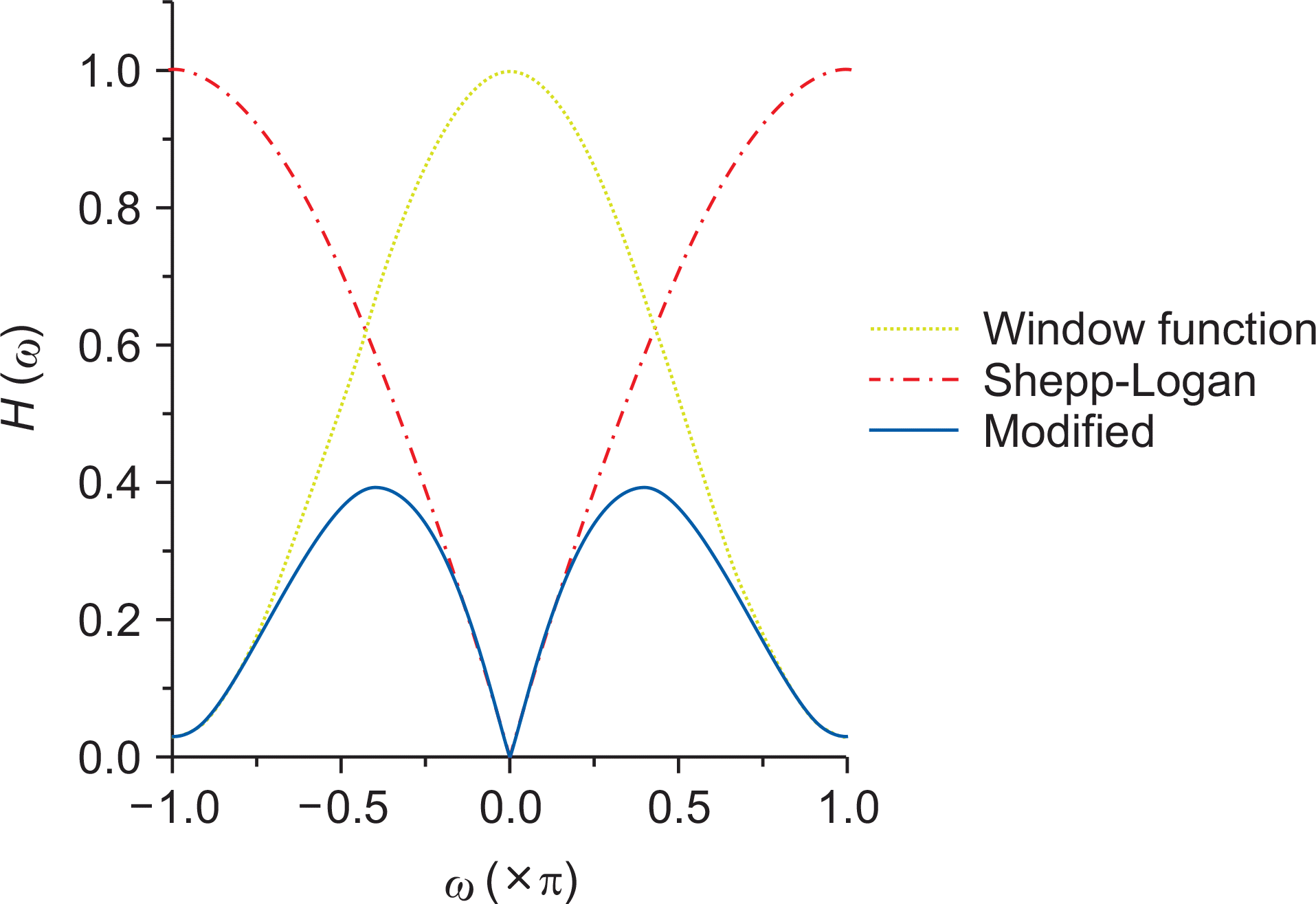
This study aims to develop an improved Feldkamp-Davis-Kress (FDK) reconstruction algorithm using anisotropic total variation (ATV) minimization to enhance the image quality of low-dose conebeam computed tomography (CBCT). The algorithm first applies a filter that integrates the Shepp-Logan filter into a cosine window function on all projections for impulse noise removal. A total variation objective function with anisotropic penalty is then minimized to enhance the difference between the real structure and noise using the steepest gradient descent optimization with adaptive step sizes. The preserving parameter to adjust the separation between the noise-free and noisy areas is determined by calculating the cumulative distribution function of the gradient magnitude of the filtered image obtained by the application of the filtering operation on each projection. With these minimized ATV projections, voxel-driven backprojection is finally performed to generate the reconstructed images. The performance of the proposed algorithm was evaluated with the catphan503 phantom dataset acquired with the use of a low-dose protocol. Qualitative and quantitative analyses showed that the proposed ATV minimization provides enhanced CBCT reconstruction images compared with those generated by the conventional FDK algorithm, with a higher contrast-to-noise ratio (CNR), lower root-mean-square-error, and higher correlation. The proposed algorithm not only leads to a potential imaging dose reduction in repeated CBCT scans via lower mA levels, but also elicits high CNR values by removing noisy corrupted areas and by avoiding the heavy penalization of striking features.
REFERENCES
1.Nabavizadeh N., Elliott DA., Chen Y, et al. Image guided radiation therapy (IGRT) practice patterns and IGRT's impact on workflow and treatment planning: Results from a national survey of American Society for Radiation Oncology members. International Journal of Radiation Oncology∗ Biology∗ Physics. 2016. 94(4):850–57.

2.Lu L., Diaconu C., Djemil T, et al. Intra–and inter–fractional liver and lung tumor motions treated with SBRT under active breathing control. Journal of Applied Clinical Medical Physics. 2018. 19(1):39–45.
3.Yoganathan S., Maria Das K., Maria Midunvaleja K, et al. Evaluating the image quality of cone beam CT acquired during rotational delivery. The British Journal of Radiology. 2015. 88(1054):20150425.

4.Alaei P., Spezi E. Imaging dose from cone beam computed tomography in radiation therapy. Physica Medica. 2015. 31(7):647–58.

5.Wang J., Li T., Liang Z., Xing L. Dose reduction for kilovotage cone-beam computed tomography in radiation therapy. Physics in Medicine and Biology. 2008. 53(11):): 2897.

6.Feldkamp LA., Davis LC., Kress JW. Practical cone-beam algorithm. Journal of the Optical Society of America A. 1984. 1(6):612–19.

7.Ahmadi R., Farahani JK., Sotudeh F., Zhaleh A., Garshasbi S. Survey of image denoising techniques. Life Science Journal. 2013. 10(1):753–55.
8.Motwani MC., Gadiya MC., Motwani RC., Harris FC. Survey of image denoising techniques. Proceedings of GSPX. 2004. 27–30.
9.Sidky EY., Pan X. Image reconstruction in circular conebeam computed tomography by constrained, total-variation minimization. Physics in Medicine and Biology. 2008. 53(17):4777–807.

10.Perona P., Malik J. Scale-space and edge detection using anisotropic diffusion. IEEE Transactions on Pattern Analysis and Machine Intelligence. 1990. 12(7):629–39.

11.Wang J., Li T., Xing L. Iterative image reconstruction for CBCT using edge–preserving prior. Medical Physics. 2009. 36(1):252–60.

12.Sidky EY., Pan X., Reiser IS., Nishikawa RM., Moore RH., Kopans DB. Enhanced imaging of microcalcifications in digital breast tomosynthesis through improved image-reconstruction algorithms. Medical Physics. 2009. 36:4920–32.

13.Lee H., Xing L., Davidi R., Li R., Qian J., Lee R. Improved compressed sensing-based cone-beam CT reconstruction using adaptive prior image constraints. Physics in Medicine and Biology. 2012. 57(8):2287–307.

14.Sun T., Sun N., Wang J., Tan S. Iterative CBCT reconstruction using Hessian penalty. Physics in Medicine and Biology. 2015. 60(5):): 1965.

15.Lee H., Fahimian BP., Xing L. Binary moving-blocker-based scatter correction in cone-beam computed tomography with width-truncated projections: proof of concept. Physics in Medicine and Biology. 2017. 62(6):): 2176.

16.Wang J., Lu H., Li T., Liang Z. Sinogram noise reduction for low-dose CT by statistics-based nonlinear filters. Medical Imaging 2005: Image Processing: International Society for Optics and Photonics. 2005. 2058–67.

Fig. 2
Example of the CDF plot generated with the gradient magnitude calculated at all the pixels of the filtered projection data.
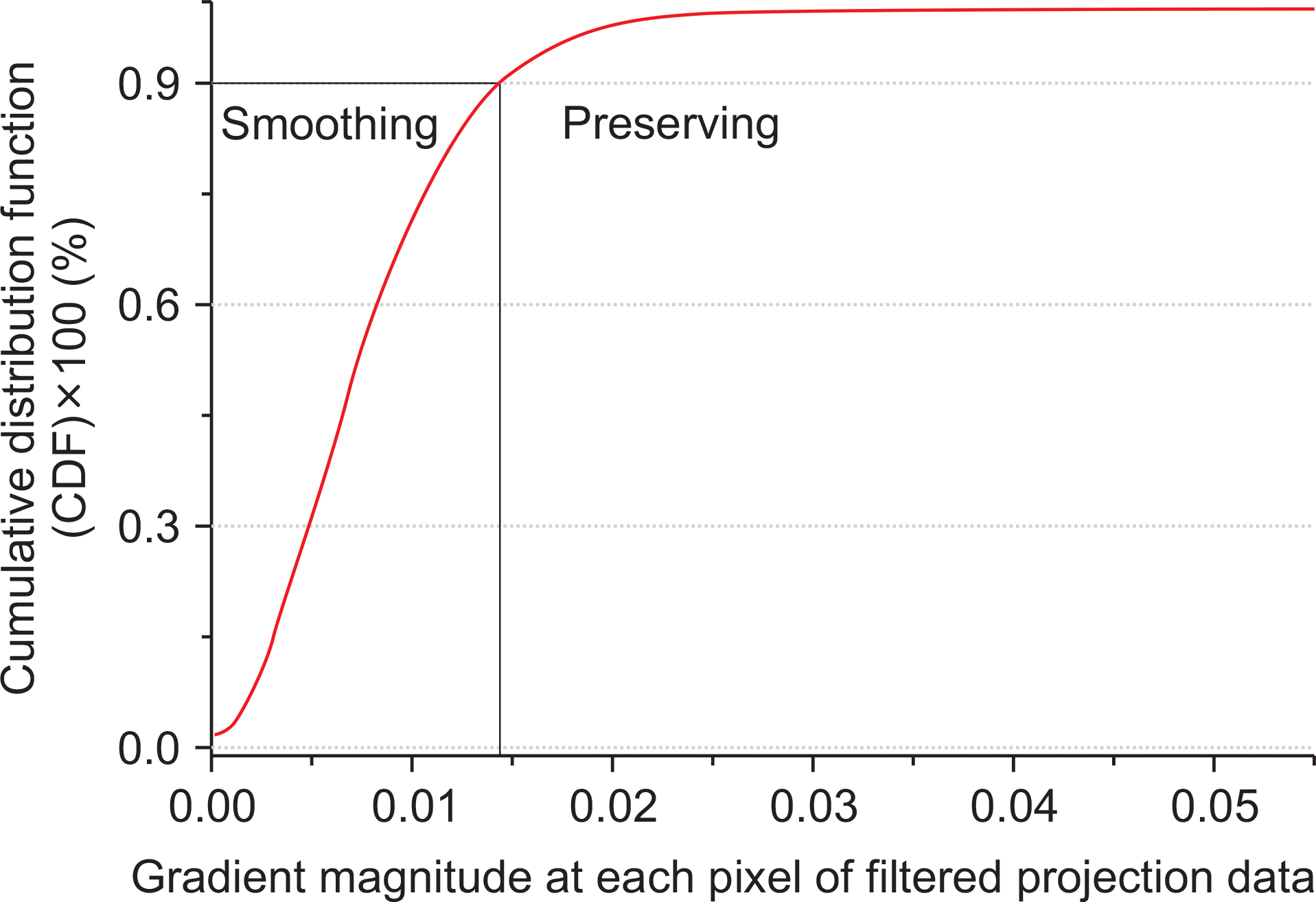
Fig. 3
Comparisons of the same views of the reconstructed image generated by applying (a) FDK with the Shepp–Logan filter, (b) FDK with a modified filter, and (c) FDK with ATV. The top row contains the CTP404 module and the bottom row contains the CTP486 module. All the images are displayed using W=1600 and L=200 HU.

Fig. 4
Comparison of reconstructed images for a representative slice generated by applying (a) conventional FDK using high-dose projections and (b) FDK with ATV using low-dose projections. These images, including the CTP528 module, are displayed using W=2400 and L=200 HU.
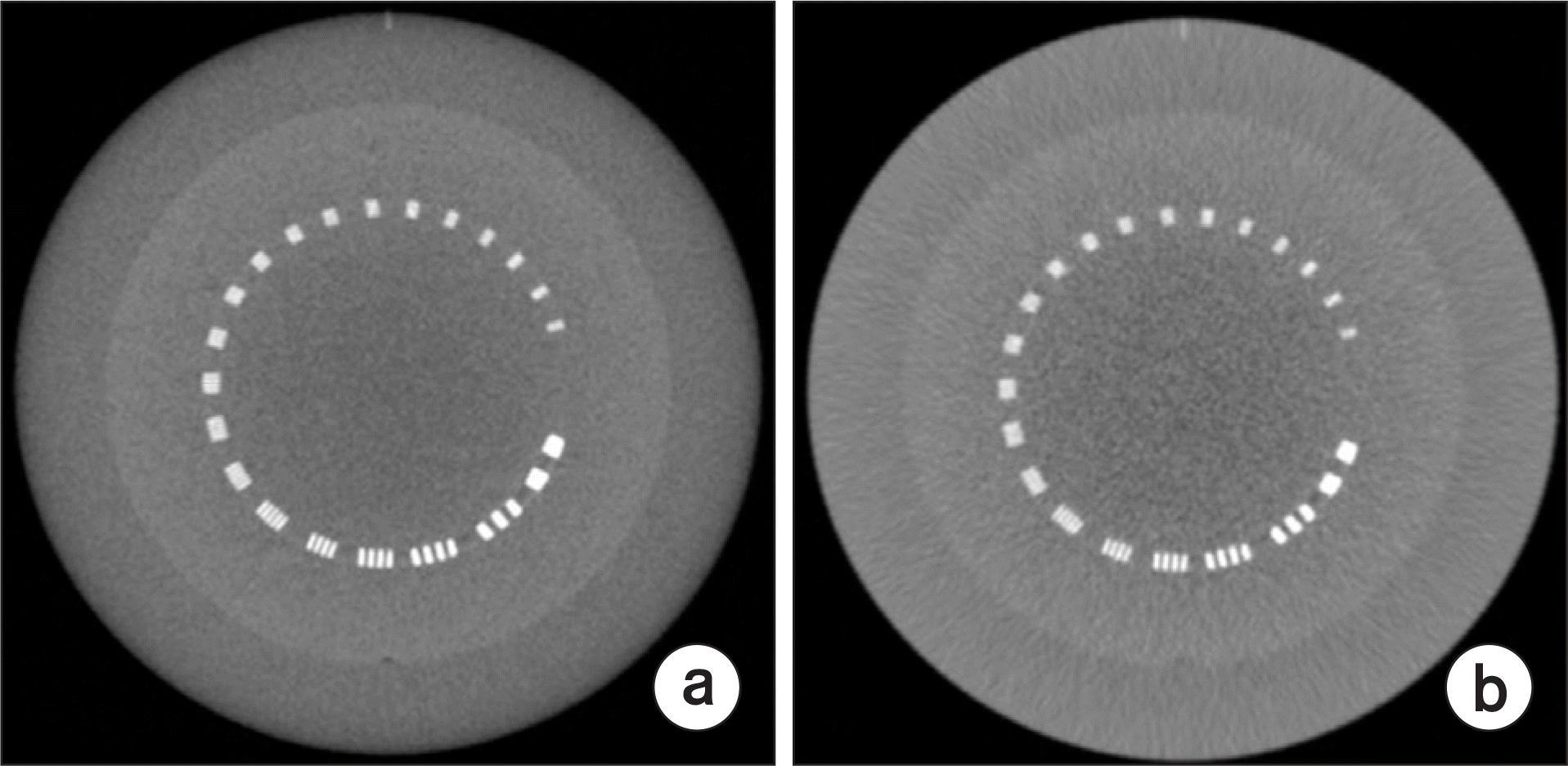
Table 1.
Comparison of contrast-to-noise rations at seven ROIs in the reconstructed images generated based on three FDK algorithms with low-dose projection data of the catphan503 phantom (CTP404).
| ROI | FDK with Shepp-Logan filter | FDK with modified filter | FDK with ATV |
|---|---|---|---|
| 1 | 3.15 | 6.29 | 11.69 |
| 2 | 5.50 | 12.94 | 23.66 |
| 3 | 4.84 | 11.59 | 23.14 |
| 4 | 0.05 | 0.48 | 1.36 |
| 5 | 0.38 | 0.81 | 1.64 |
| 6 | 0.87 | 1.58 | 2.76 |
| 7 | 5.14 | 11.64 | 23.09 |




 PDF
PDF ePub
ePub Citation
Citation Print
Print


 XML Download
XML Download