1. Gjessing P. Controlled retraction of maxillary incisors. Am J Orthod Dentofacial Orthop. 1992; 101:120–131.

2. Park YC, Choy K, Lee JS, Kim TK. Lever-arm mechanics in lingual orthodontics. J Clin Orthod. 2000; 34:601–605.
3. Chung KR, Oh MY, Ko SJ. Corticotomy-assisted orthodontics. J Clin Orthod. 2001; 35:331–339.
4. Hong RK, Heo JM, Ha YK. Lever-arm and mini-implant system for anterior torque control during retraction in lingual orthodontic treatment. Angle Orthod. 2005; 75:129–141.
5. Kim SH, Park YG, Chung K. Severe Class II anterior deep bite malocclusion treated with a C-lingual retractor. Angle Orthod. 2004; 74:280–285.
6. Park YC, Chu JH, Choi YJ, Choi NC. Extraction space closure with vacuum-formed splints and miniscrew anchorage. J Clin Orthod. 2005; 39:76–79.
7. Chung KR, Cho JH, Kim SH, Kook YA, Cozzani M. Unusual extraction treatment in Class II division 1 using C-orthodontic mini-implants. Angle Orthod. 2007; 77:155–166.

8. Park YC, Choi YJ, Choi NC, Lee JS. Esthetic segmental retraction of maxillary anterior teeth with a palatal appliance and orthodontic mini-implants. Am J Orthod Dentofacial Orthop. 2007; 131:537–544.

9. Chung KR, Kook YA, Kim SH, Mo SS, Jung JA. Class II malocclusion treated by combining a lingual retractor and a palatal plate. Am J Orthod Dentofacial Orthop. 2008; 133:112–123.

10. Jang HJ, Roh WJ, Joo BH, Park KH, Kim SJ, Park YG. Locating the center of resistance of maxillary anterior teeth retracted by Double J Retractor with palatal miniscrews. Angle Orthod. 2010; 80:1023–1028.

11. Lee EH, Yu HS, Lee KJ, Park YC. Three dimensional finite element analysis of continuous and segmented arches with use of orthodontic miniscrews. Korean J Orthod. 2011; 41:237–254.

12. Park JH, Tai K, Takagi M, Miyajima K, Kojima Y, Joo BH. Esthetic orthodontic treatment with a double J retractor and temporary anchorage devices. Am J Orthod Dentofacial Orthop. 2012; 141:796–805.

13. Mo SS, Kim SH, Sung SJ, Chung KR, Chun YS, Kook YA, et al. Torque control during lingual anterior retraction without posterior appliances. Korean J Orthod. 2013; 43:3–14.

14. Osipenko MA, Nyashin NY, Nyashin YI. Center of resistance and center of rotation of a tooth: the definitions, conditions of existence, properties. Russ J Biomech. 1999; 1:5–15.
15. Kojima Y, Kawamura J, Fukui H. Finite element analysis of the effect of force directions on tooth movement in extraction space closure with miniscrew sliding mechanics. Am J Orthod Dentofacial Orthop. 2012; 142:501–508.

16. Kojima Y, Fukui H. A finite element simulation of initial movement, orthodontic movement, and the centre of resistance of the maxillary teeth connected with an archwire. Eur J Orthod. 2014; 36:255–261.

17. Kojima Y, Fukui H. Numerical simulations of canine retraction with T-loop springs based on the updated moment-to-force ratio. Eur J Orthod. 2012; 34:10–18.

18. Kim MJ, Park JH, Kojima Y, Tai K, Chae JM. A finite element analysis of the optimal bending angles in a running loop for mesial translation of a mandibular molar using indirect skeletal anchorage. Orthod Craniofac Res. 2018; 21:63–70.





 PDF
PDF ePub
ePub Citation
Citation Print
Print




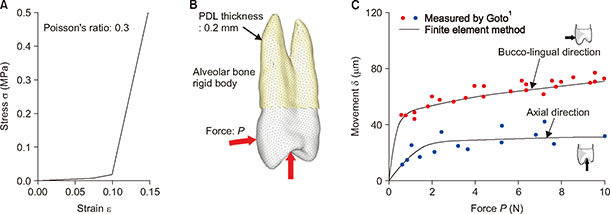
 XML Download
XML Download