Abstract
Purpose
To evaluate and compare published methods of calculating intraocular lens (IOL) power following myopic laser refractive surgery.
Methods
We performed a retrospective review of the medical records of 69 patients (69 eyes) who had undergone myopic laser refractive surgery previously and subsequently underwent cataract surgery at Samsung Medical Center in Seoul, South Korea from January 2010 to June 2016. None of the patients had pre-refractive surgery biometric data available. The Haigis-L, Shammas, Barrett True-K (no history), Wang-Koch-Maloney, Scheimpflug total corneal refractive power (TCRP) 3 and 4 mm (SRK-T and Haigis), Scheimpflug true net power, and Scheimpflug true refractive power (TRP) 3 mm, 4 mm, and 5 mm (SRK-T and Haigis) methods were employed. IOL power required for target refraction was back-calculated using stable post-cataract surgery manifest refraction, and implanted IOL power and formula accuracy were subsequently compared among calculation methods.
Results
Haigis-L, Shammas, Barrett True-K (no history), Wang-Koch-Maloney, Scheimpflug TCRP 4 mm (Haigis), Scheimpflug true net power 4 mm (Haigis), and Scheimpflug TRP 4 mm (Haigis) formulae showed high predictability, with mean arithmetic prediction errors and standard deviations of −0.25 ± 0.59, −0.05 ± 1.19, 0.00 ± 0.88, −0.26 ± 1.17, 0.00 ± 1.09, −0.71 ± 1.20, and 0.03 ± 1.25 diopters, respectively.
Conclusions
Visual outcomes within 1.0 diopter of target refraction were achieved in 85% of eyes using the calculation methods listed above. Haigis-L, Barrett True-K (no history), and Scheimpflug TCRP 4 mm (Haigis) and TRP 4 mm (Haigis) methods showed comparably low prediction errors, despite the absence of historical patient information.
A significant number of patients have undergone laser refractive surgery over the past decade. Furthermore, millions of postrefractive surgery patients are currently at an age at which senile cataracts can develop [1]. When patients who have undergone laser refractive surgery later have cataract surgery, they often have high expectations for emmetropia as they did with their prior refractive surgery procedure [2]. Unfortunately, however, intraocular lens (IOL) calculation after laser refractive surgery is more difficult and less accurate than when calculated for the original cornea [34]. In particular, “hyperopic surprise” in post-myopic laser refractive surgery patients is common.
The three main reasons for this IOL calculation inaccuracy are error in corneal radius measurement, keratometric (K) index error, and error in the IOL power calculation [5]. To compensate for posterior corneal curvature, traditional keratometers and topographers use a standardized index of refraction to convert measurements of anterior corneal curvature to refractive power of the entire cornea. In most devices, a value of 1.3375 is used. However, because ablative corneal refractive surgery alters the relationship between the front and back surfaces of the cornea, the use of a standardized index of refraction of 1.3375 is no longer valid in such cases. This problem can be overcome by the development of devices that directly and accurately measure anterior and posterior corneal curvature or, perhaps preferably, actual corneal refractive power. One currently available technology is Scheimpflug imaging. To date, numerous methods have been introduced to minimize and compensate for error in the post-refractive surgery IOL calculation [678]. These methods can be divided into those that employ the patient's clinical history data and those that do not. Historically, IOL calculation formulae that included clinical history method were the gold-standard methods. However, methods that do not use clinical history, which include Haigis-L [9], Shammas [10], Barrett True-K (no history) [111213], and Wang-Koch-Maloney (WKM) methods [14] have shown improved accuracy in comparison with traditional clinical history methods. These methods do not require historical information; instead, they rely on indices such as current biometry, keratometer index change, and K correction factor. As such, it is very important when employing these techniques to accurately measure the patient's actual corneal power after laser refractive surgery for accurate IOL calculation.
With this in mind, our purpose in the current study was to evaluate and compare previously published methods with methods that employ biometric data measured using a Scheimpflug (Pentacam HR; Oculus, Wetzlar, Germany) system with respect to the efficacy of IOL power calculations after myopic laser refractive surgery. In addition, we sought to provide guidelines for selecting the best method for IOL calculation in eyes without clinical history data.
We sought to compare several K values or corneal radii from the Scheimpflug system to find the most accurate K value for IOL power calculations. Additionally, we sought to compare the accuracy of various IOL calculation methods in the same patients to determine which formula is the most accurate for calculating IOL power in cataract patients after corneal refractive surgery.
We performed a retrospective review of the medical records of 69 patients (69 eyes) who underwent myopic laser refractive surgery and subsequent cataract surgery at Samsung Medical Center in Seoul, South Korea from January 2010 to June 2016. The study was completed in accordance with the principles of the Declaration of Helsinki and was approved by the institutional review board of Samsung Medical Center (2018-11-140) and informed consent was waived by the board.
All surgeries were carried out by one of two experienced surgeons (TYC and ESC) using the same technique of clear corneal incision, phacoemulsification, and implantation of the IOL(s) in the capsular bag. The patients had no pre-refractive surgery biometric data and were examined prior to cataract surgery, undergoing a thorough ophthalmologic inspection including slit-lamp examination, visual acuity, manifest refraction, potential acuity meter, and optical biometry. Biometry measured on the date closest to cataract surgery was used to calculate IOL power. Partial coherence interferometry (PCI) (Zeiss IOLMaster; Carl Zeiss Meditec, Dublin, CA, USA) measurements were used in this study. All patients underwent biometry of axial length (AL), anterior chamber depth, and K measurements with PCI. Haigis-L, Shammas, WKM, and Barrett True-K (no history) formulae were used to calculate IOL power using online calculators provided by the ASCRS (http://iolcalc.ascrs.org/wbfrmCalculator.aspx) and APASCRS (http://www.apacrs.org/barrett_true_K_universal_2).
In addition, K data for IOL calculations for cataract surgery were obtained using values obtained from Scheimpflug system's total corneal refractive power (TCRP) map, true net corneal power (TNP) map, and total refractive power (TRP) map. K values were obtained for several different zones (3, 4, and 5 mm) in each map (TCRP, TNP, and TRP). PCI was used for AL calculations. SRK/T and the Haigis formula was used to calculate IOL power.
One of two different types of IOLs was implanted: 26 patients received a Tecnis 1-piece monofocal IOL and 30 patients received an AcrySof IQ monofocal IOL (Alcon, Fort Worth, TX, USA).
In total, 20 formulae (Haigis-L; Shammas; WKM; Barrett True-K; TCRP 3 and 4 mm [SRK-T, Haigis]; TNP 3, 4, and 5 mm [SRK-T, Haigis]; and TRP 3, 4, and 5 mm [SRK-T, Haigis]) were used to estimate corneal power or adjusted IOL power in patients who had previously undergone laser refractive surgery. Manifest refraction after cataract surgery was obtained for each subject by an examination three months after surgery. Then, prediction error (PE) was calculated by subtracting the predicted refraction from the power of the actual refraction. Mean numeric error, mean absolute error, and percentage of eyes within a refractive PE of ±0.5 and ±1.0 diopters (D) were calculated for each method. To assess whether the mean numeric and absolute PEs produced by various methods were significantly different, the variances of the mean numeric and absolute PEs were tested using the F-test. Bonferroni correction was applied for multiple tests. Statistical analyses were performed using Microsoft Excel 2007 (Microsoft Corp., Redmond, WA, USA) and IBM SPSS Statistics ver. 22 (IBM Corp., Armonk, NY, USA). Statistical significance was defined as p < 0.05.
Cases were divided into two groups according to their axial length (≤26.00, >26.00 mm), anterior chamber depth (≤3.5, >3.5 mm), or K reading (≤39.73, >39.73). In each subgroup, mean numeric error, mean absolute error, and the percentage of eyes within a refractive PE of ±0.5 and ±1.0 D were calculated for each method.
Of the 69 patients selected, 56 patients (56 eyes) who underwent myopic laser refractive surgery and subsequent cataract surgery were included in this study. Mean age of the patients included was 54.6 ± 9.37 years; 21 (37.5%) were male and 35 (62.5%) were female. Among the 56 eyes, 30 (53.6%) eyes were right eyes and 26 (46.4%) eyes were left eyes. Mean best-corrected visual acuity before cataract surgery was 0.34 ± 0.23 according to the logarithm of the minimum angle of resolution (logMAR) scale. Mean spherical equivalent before cataract surgery was −2.83 ± 3.52 D. Mean AL as measured by PCI was 27.04 ± 2.36 mm, mean K value using PCI before cataract surgery was 39.73 ± 2.33 D, and mean anterior chamber depth was 3.63 ± 0.34 mm (Table 1).
Mean IOL power was 19.63 ± 2.67 D. Mean uncorrected visual acuity and best-corrected visual acuity 3 months after cataract surgery were 0.26 ± 0.33 and 0.05 ± 0.08 log-MAR, respectively. Mean spherical equivalent and astigmatism 3 months after cataract surgery were −1.32 ± 1.32 and −0.61 ± 0.49 D, respectively (Table 1). Emmetropia or −0.5 D was aimed for in most patients but in some patients the target was a diopter value near (−2.5 to −3.0 D) or similar to that of their opposite eye (e.g., −5.25 D).
Comparison of the 20 different formulas revealed that the accuracy of the IOL calculation was more precise in cases using the Haigis formula with K values of the Scheimpflug system rather than those using the SRK/T formula (Appendix 1). Therefore, in this study, Scheimpflug system formulae (TCRP, TNP, and TRP) using the Haigis formula were compared with previous formulas. Specifically, mean numeric PEs and standard deviations of the Haigis-L, Shammas, Barrett True-K (no history), WKM, TCRP 4 mm K (Haigis), TNP 4 mm K (Haigis), and TRP 4 mm K (Haigis) formulae were −0.25 ± 0.59, −0.05 ± 1.19, 0.00 ± 0.88, −0.26 ± 1.17, 0.00 ± 1.09, −0.71 ± 1.20, and 0.03 ± 1.25 D, respectively (Fig. 1).
In terms of absolute PEs and standard deviations of the Haigis-L, Shammas, Barrett True-K (no history), WKM, TCRP 4 mm K (Haigis), TNP 4 mm K (Haigis), and TRP 4 mm K (Haigis) formulae, values were 0.51 ± 0.44, 0.92 ± 0.74, 0.00 ± 0.88, 0.94 ± 0.74, 0.82 ± 0.7, 1.16 ± 0.77, and 0.89 ± 0.86 D, respectively (Fig. 2). The four most accurate corneal power adjustment and formula combinations were the Haigis-L, Barrett True-K (no history), TCRP 4 mm K (Haigis), and TRP 4 mm K (Haigis). Numeric PE and absolute PE as measured with the Haigis-L and Barrett True-K (no history) methods were lower than those measured with the TCRP 4 mm K (Haigis) and TRP 4 mm K (Haigis) methods; however, the differences were not statistically significant (p = 0.241 and p = 0.361, respectively). There were no statistically significant differences in numeric PE or absolute PE according to type of IOL (p = 0.357 and p = 0.293) or surgeon (p = 0.264 and p = 0.328).
The percentage of individuals in which each formula combination predicted between ±0.5 and ±1.0 D relative to the target refraction was also evaluated (Fig. 3). The Haigis-L, Shammas, Barrett True-K (no history), WKM, TCRP 4 mm K (Haigis), TNP 4 mm K (Haigis), and TRP 4 mm K (Haigis) percentages were 58.9%, 37.5%, 57.4%, 33.9% 42.6%, 18.5%, and 31.5% within ±0.5 D and 85.7%, 58.9%, 75.9%, 55.4% 70.4%, 44.4%, and 72.2% within ±1.0 D of the refractive PE, respectively. These results indicated that the four most accurate corneal power adjustment techniques and formula combinations were the Haigis-L, Barrett True-K (no history), TCRP 4 mm K (Haigis), and TRP 4 mm K (Haigis).
Cases were divided into two groups according to axial length (≤26.00, >26.00 mm), K (≤39.73, >39.73), or anterior chamber depth (≤3.5, >3.5 mm). Each subgroup was also evaluated in terms of numeric PEs, absolute PEs, and the number of eyes for which each formula's prediction was within ±0.5 D, and ±1.0 D of the actual refraction after surgery. Among subgroups, only the subgroup designated based on axial length showed a significant difference in numeric and absolute PEs. Haigis-L (p = 0.021, 0.017), Barrett True-K (no history; p = 0.031, 0.028), TCRP 4 mm K (Haigis; p = 0.041, 0.033), and TRP 4 mm K (Haigis; p = 0.037, 0.029) formulae were less accurate for those eyes with a longer axial length (Appendix 2, 3, 4).
Calculation of corneal power and IOL power for patients who have undergone laser refractive surgery remains a challenge. In an effort to address this, the British National Health Service (NHS) proposed two benchmark standards for refractive outcomes following cataract surgery: that 55% of normal eyes be within ±0.5 D and 85% of normal eyes be within ±1.0 D of the targeted spherical equivalent [15]. In a previous study, McCarthy et al. [7] reported that only the Masket and Masket [16] formula satisfied the first of the NHS benchmark standards, while none of the techniques satisfied the second NHS benchmark standard that 85% of eyes be within ±1.0 D of the target refraction. Furthermore, the reported values within ±0.5 and ±1.0 D remain highly variable among studies [17].
The Maloney and WKM methods recalculate postoperative K values to the exact power present at the anterior corneal surface and then add an average negative power value for the posterior corneal surface [14]. The Pentacam system uses a rotating Scheimpflug camera to measure both the anterior and posterior corneal curvatures and offers at least two options to overcome this issue: 1) corneal power calculated by ray tracing (TCRP) and 2) corneal power calculated by the Gaussian optics formula (TNP). Both the former and to a lesser extent the latter have been found to accurately track the refractive change induced by myopic excimer laser surgery [1819]. To overcome the corneal power measurement error, Holladay et al. [20] recommended using equivalent K readings and Savini et al. [19] and Savini et al. [21] reported corneal power determination by the ray-tracing method (TCRP) using the Pentacam. Savini et al. [21] reported that the TCRP 3-mm zone accurately reflected the surgically-induced corneal change in power. More recently, corneal power and IOL calculation methods based on Fourier-domain optical coherence tomography (OCT) have been reported [2223]. The RTVue (Optovue, Fremont, CA, USA), which is based on spectral-domain OCT (SD-OCT), was introduced to calculate IOL power after corneal refractive surgery [2223]. However, this method has limitations; among all SD-OCT devices, only RTVue provides this method, and because of motion artifacts, it can only perform ray-tracing in the paraxial limit. Additionally, the OCT formula itself requires complicated calculations because of the nature of the regression formula. So, while the results obtained are comparably accurate, SD-OCT is still not a common device for corneal power and IOL calculations.
Unlike previous studies that predominantly calculated IOL formulae using single corneal power (TNP) measured with the Pentacam system, we evaluated various different corneal K readings such as TCRP, TNP, and TRP, and included several zones (3, 4, 5 mm) [242526]. Furthermore, we used the Haigis formula to overcome the limitations of third-generation formulae. If postoperative corneal power is used to calculate effective lens position, then the calculated lens position will be more anterior than what is true. This will result in a target IOL of insufficient power. Hence, we compared both SRK/T and Haigis formulae for each K reading and found that the Haigis formula was more accurate than the SRK-T formula in most cases (Appendix 1).
Kim et al. [24] compared the accuracy of IOL power predictions using the Orbscan II topography system (Bausch & Lomb; Orbtek, Salt Lake City, UT, USA), IOLMaster, and Pentacam system and reported that the TNP in the latter system may be relatively predictable as compared with other K values. However, in their study, TNP calculated using the SRK-T formula resulted in 31.3% of eyes that were within ±0.5 D PE of actual postoperative refraction and 51.7% of eyes that were within ±1.0 D PE of actual postoperative diffraction. In our study, the most accurate formulae using corneal power as determined with the Pentacam were TCRP 4 mm and TRP 4 mm with Haigis. Notably, our results were more accurate than those of Kim et al. [24]. In a separate study, Kim et al. [25] found that the TNP of the Pentacam system provided an accurate K reading for calculating IOL power in postoperative refractive surgery eyes. In this study, the actual refraction was within ±0.5 D of the intended refraction for 70% of eyes (21 / 30) and within ±1.0 D for 93% of eyes (28 / 30). However, our sample size was relatively small at only 30 eyes.
In our study, the IOL calculation accuracy of 20 formulae (Haigis-L, Shammas, Barrett True-K [no history], WKM, TCRP 3 and 4 mm [SRK-T, Haigis], TNP 3, 4, and 5 mm [SRK-T, Haigis], and TRP 3, 4, and 5 mm [SRK-T, Haigis]) were analyzed and compared. The best K readings as measured using the Pentacam system were with TCRP 4 mm and TRP 4 mm. Especially, the TCRP (4 mm, Haigis) and TRP (4 mm, Haigis) methods provided comparably accurate IOL predictions for numeric PE (0.00 ± 1.09 and 0.03 ± 1.25 D, respectively), absolute PE (0.82 ± 0.7 and 0.89 ± 0.86 D, respectively), and the percentage of eyes within ±0.5 D (42.6% and 31.5%, respectively) and ±1.0 D (70.4% and 72.2%, respectively) of the refractive PE. Furthermore, there were no significant differences between the Haigis-L and Barrett True-K methods (p = 0.241 and p = 0.361, respectively).
In our study, additional subgroup analysis revealed that using the same formula, IOL calculations for eyes with relatively long axial length and low corneal power (K) tended to be less accurate. This is important for especially long eyes, which are more likely to undergo refractive surgery because of myopia.
Limitations of this study include the use of different IOL models, specifically the Tecnis and AcrySof IQ monofocal IOL. However, as can be seen from the mean numeric and absolute PE calculations, there was no statistically significant differences between the two different IOLs (p = 0.357 and p = 0.293). Another limitation is that the surgeries were performed by two different experienced surgeons (TYC and ESJ); nevertheless, in subgroup analysis, there were no statistically significant differences in numeric and absolute PE values between the two surgeons (p = 0.264 and p = 0.328). Last, our study enrolled patients who underwent myopic refractive surgery with a mean axial length and anterior chamber depth of 27.08 and 3.63 mm, respectively. Patients with short axial length and shallow anterior chamber were therefore underrepresented in our study.
We analyzed 20 formula combinations in our study. The most accurate method was the Haigis-L, with 85.7% of eyes within ±1.0 D of target refraction. TCRP (4 mm, Haigis) and TRP (4 mm, Haigis) predicted between 70.4% and 72.2% of eyes within ±1.0 D of target refraction without the use of prior clinical data. Only the Haigis-L and Barrett True-K (no history) methods were more accurate than TCRP and TRP among the 20 formulae. With regard to measurement of corneal power using the Pentacam, TCRP 4 mm (Haigis) and TRP 4 mm (Haigis) may possibly be useful for corneal power calculations and IOL power calculations in post-refractive surgery patients with no pre-operative clinical data available. Additional research is needed to improve the accuracy of IOL calculation methods based on regression formulae to modify corneal power using the Pentacam system (TCRP and TRP) with the Haigis formula.
Figures and Tables
 | Fig. 1Mean numeric prediction error (PE). A comparison of mean arithmetic PE between the Haigis-L, Shammas, Barrett True-K, and Wang-Koch-Maloney (WKM) methods with Haigis formulas using input values from the Pentacam system. The most accurate corneal power adjustment and formula combinations were the Haigis-L, Barrett True-K, and total corneal refractive power (TCRP) 4 mm (Haigis). TNP = true net power; TRP = total refractive power. |
 | Fig. 2Mean absolute prediction error. A comparison of mean absolute prediction error values calculated with the Haigis-L, Shammas, Barrett True-K, and Wang-Koch-Maloney (WKM) with the Haigis formulae using values obtained with the Pentacam system. The most accurate corneal power adjustment and formula combinations were the Haigis-L, Barrett True-K, and total corneal refractive power (TCRP) 4 mm (Haigis). TNP = true net power; TRP = total refractive power. |
 | Fig. 3Percentages of eyes within ±0.5 and ±1.0 diopters (D) of the target refraction. A comparison of the percentages of eyes within ±0.5 and ±1.0 D of the target refraction for the difference formulae (the Haigis-L, Shammas, Barrett True-K, and Wang-Koch-Maloney [WKM] with Haigis formulae) using values obtained from the Pentacam system. The most accurate corneal power adjustment and formula combinations were the Haigis-L, Barrett True-K, and total corneal refractive power (TCRP) 4 mm (Haigis) and total refractive power (TRP) 4 mm (Haigis). TNP = true net power. |
Table 1
Preoperative patient demographics of the initial 69 patients (69 eyes) enrolled before cataract surgery

Values are presented as mean ± standard deviation (range) or number.
OD = right eye; OS = left eye; UCVA = uncorrected visual acuity; logMAR = logarithm of the minimum angle of resolution; BCVA = best-corrected visual acuity; D = diopter; PCI = partial coherence interferometry; ACD = anterior chamber depth; K = keratometry.
Appendices
Appendix 1
Numeric and absolution PE values and percentages of eyes within ±0.5 and ±1.0 D of the target refraction for all 20 methods

PE = prediction error; D = diopter; TCRP = total corneal refractive power; TNP = true net power; TRP = total refractive power.
*Mean arithmetic PE; †Mean absolute PE; ‡,§Percentages of eyes within ±0.5 and ± 1.0 D of the target refraction.
Appendix 2
Numeric, absolute PEs, and percentages of eyes within ±0.5 and ±1.0 D of the target refraction for the four best methods according to axial length
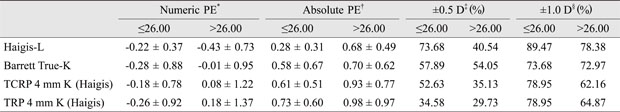
PE = prediction error; D = diopter; TCRP = total corneal refractive power; TRP = total refractive power.
*Mean arithmetic PE; †Mean absolute PE; ‡,§Percentages of eyes within ±0.5 and ± 1.0 D of the target refraction.
Appendix 3
Numeric, absolute PEs, and percentages of eyes within ±0.5 and ±1.0 D of target refraction for the best four methods according to anterior chamber depth
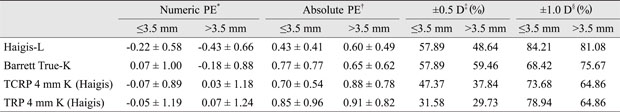
PE = prediction error; D = diopter; TCRP = total corneal refractive power; TRP = total refractive power.
*Mean arithmetic PE; †Mean absolute PE; ‡,§Percentages of eyes within ±0.5 and ± 1.0 D of the target refraction.
Appendix 4
Numeric, absolute PEs, and percentages of eyes within ±0.5 and ±1.0 D of the target refraction for the best four methods according to K subgroup

PE = prediction error; D = diopter; TCRP = total corneal refractive power; TRP = total refractive power.
*Mean arithmetic PE; †Mean absolute PE; ‡,§Percentages of eyes within ±0.5 and ± 1.0 D of the target refraction.
References
1. Klein BE, Klein R, Lee KE, Gangnon RE. Incidence of age-related cataract over a 15-year interval the Beaver Dam Eye Study. Ophthalmology. 2008; 115:477–482.
2. Koch DD. New options for IOL calculations after refractive surgery. J Cataract Refract Surg. 2006; 32:371–372.

3. Seitz B, Langenbucher A, Nguyen NX, et al. Underestimation of intraocular lens power for cataract surgery after myopic photorefractive keratectomy. Ophthalmology. 1999; 106:693–702.

4. Seitz B, Langenbucher A. Intraocular lens power calculation in eyes after corneal refractive surgery. J Refract Surg. 2000; 16:349–361.

5. Hoffer KJ. Intraocular lens power calculation after previous laser refractive surgery. J Cataract Refract Surg. 2009; 35:759–765.

6. Wang L, Hill WE, Koch DD. Evaluation of intraocular lens power prediction methods using the American Society of Cataract and Refractive Surgeons Post-Keratorefractive In traocular Lens Power Calculator. J Cataract Refract Surg. 2010; 36:1466–1473.
7. McCarthy M, Gavanski GM, Paton KE, Holland SP. Intraocular lens power calculations after myopic laser refractive surgery: a comparison of methods in 173 eyes. Ophthalmology. 2011; 118:940–944.

8. Savini G, Barboni P, Carbonelli M, et al. Intraocular lens power calculation after myopic excimer laser surgery: Selecting the best method using available clinical data. J Cataract Refract Surg. 2015; 41:1880–1888.

9. Haigis W. Intraocular lens calculation after refractive surgery for myopia: Haigis-L formula. J Cataract Refract Surg. 2008; 34:1658–1663.

10. Shammas HJ, Shammas MC. No-history method of intraocular lens power calculation for cataract surgery after myopic laser in situ keratomileusis. J Cataract Refract Surg. 2007; 33:31–36.

11. Barrett GD. Intraocular lens calculation formulas for new intraocular lens implants. J Cataract Refract Surg. 1987; 13:389–396.

12. Barrett GD. An improved universal theoretical formula for intraocular lens power prediction. J Cataract Refract Surg. 1993; 19:713–720.

13. Abulafia A, Hill WE, Koch DD, et al. Accuracy of the Barrett True-K formula for intraocular lens power prediction after laser in situ keratomileusis or photorefractive keratectomy for myopia. J Cataract Refract Surg. 2016; 42:363–369.

14. Wang L, Booth MA, Koch DD. Comparison of intraocular lens power calculation methods in eyes that have undergone LASIK. Ophthalmology. 2004; 111:1825–1831.

15. Gale RP, Saldana M, Johnston RL, et al. Benchmark standards for refractive outcomes after NHS cataract surgery. Eye (Lond). 2009; 23:149–152.

16. Masket S, Masket SE. Simple regression formula for intraocular lens power adjustment in eyes requiring cataract surgery after excimer laser photoablation. J Cataract Refract Surg. 2006; 32:430–434.

17. Hodge C, McAlinden C, Lawless M, et al. Intraocular lens power calculation following laser refractive surgery. Eye Vis (Lond). 2015; 2:7.

18. Wang L, Mahmoud AM, Anderson BL, et al. Total corneal power estimation: ray tracing method versus gaussian optics formula. Invest Ophthalmol Vis Sci. 2011; 52:1716–1722.

19. Savini G, Hoffer KJ, Carbonelli M, Barboni P. Scheimpflug analysis of corneal power changes after myopic excimer laser surgery. J Cataract Refract Surg. 2013; 39:605–610.

20. Holladay JT, Hill WE, Steinmueller A. Corneal power measurements using scheimpflug imaging in eyes with prior corneal refractive surgery. J Refract Surg. 2009; 25:862–868.

21. Savini G, Barboni P, Carbonelli M, Hoffer KJ. Comparison of methods to measure corneal power for intraocular lens power calculation using a rotating Scheimpflug camera. J Cataract Refract Surg. 2013; 39:598–604.

22. Tang M, Li Y, Huang D. An intraocular lens power calculation formula based on optical coherence tomography: a pilot study. J Refract Surg. 2010; 26:430–437.

23. Wang L, Tang M, Huang D, et al. Comparison of newer intraocular lens power calculation methods for eyes after corneal refractive surgery. Ophthalmology. 2015; 122:2443–2449.

24. Kim EC, Cho K, Hwang HS, et al. Intraocular lens prediction accuracy after corneal refractive surgery using K values from 3 devices. J Cataract Refract Surg. 2013; 39:1640–1646.

25. Kim SW, Kim EK, Cho BJ, et al. Use of the pentacam true net corneal power for intraocular lens calculation in eyes after refractive corneal surgery. J Refract Surg. 2009; 25:285–289.




 PDF
PDF ePub
ePub Citation
Citation Print
Print


 XML Download
XML Download