Abstract
Objective
The aim of this study was to determine the optimal loading conditions for pure intrusion of the six maxillary anterior teeth with miniscrews according to alveolar bone loss.
Methods
A three-dimensional finite element model was created for a segment of the six anterior teeth, and the positions of the miniscrews and hooks were varied after setting the alveolar bone loss to 0, 2, or 4 mm. Under 100 g of intrusive force, initial displacement of the individual teeth in three directions and the degree of labial tilting were measured.
Results
The degree of labial tilting increased with reduced alveolar bone height under the same load. When a miniscrew was inserted between the two central incisors, the amounts of medial-lateral and anterior-posterior displacement of the central incisor were significantly greater than in the other conditions. When the miniscrews were inserted distally to the canines and an intrusion force was applied distal to the lateral incisors, the degree of labial tilting and the amounts of displacement of the six anterior teeth were the lowest, and the maximum von Mises stress was distributed evenly across all the teeth, regardless of the bone loss.
Conclusions
Initial tooth displacement similar to pure intrusion of the six maxillary anterior teeth was induced when miniscrews were inserted distal to the maxillary canines and an intrusion force was applied distal to the lateral incisors. In this condition, the maximum von Mises stresses were relatively evenly distributed across all the teeth, regardless of the bone loss.
Periodontal disease is common in adult dentition. As inflammatory phenomena that are multifactorial in origin can destroy periodontal tissue, some sequelae may occur, including elongation, tipping, and pathologic tooth migration.1 As a result of pathologic tooth migration, occlusal trauma and periodontitis are aggravated, resulting in greater losses of attachment, extrusion, and mobility of the displaced teeth.23 These problems are particularly likely to occur in the maxillary anterior region, because these teeth are not protected by occlusal forces and have no anterior-posterior contacts to inhibit tooth migration.
To solve these problems, orthodontic treatments for the intrusion of anterior teeth are necessary in most cases.4 Miniscrews, which serve as effective temporary anchorage devices, have recently acquired an important role in the facilitation of various tooth movements, because anchorage control and patient cooperation are very critical in the field of orthodontics. Many authors have described methods involving miniscrews for the intrusion of incisors, and have reported significant amounts of incisor intrusion.567 Direct intrusion methods using miniscrews have the advantage of being able to move the teeth effectively, because they form a force vector in the desired direction without the loss of anchorage. However, the orthodontic force applied by the miniscrew is an external force in the context of the entire force system. In other words, the direction of the force strongly influences the movement pattern of the teeth.8 To move the teeth into a desired pattern, the appropriate force direction must be selected. Therefore, the relationship between the force direction and the movement pattern must be accurately clarified.
Orthodontists dealing with periodontally compromised dentitions need to understand how altered biomechanics due to reduced periodontal tissue affect their treatments.910 For example, the center of resistance (CR) in a periodontally compromised tooth moves to a more apical position, which requires that the moment/force ratio be adjusted.11 When periodontally compromised patients undergo orthodontic treatments, tooth movements should be as well-targeted and well-controlled as possible, and involve the application of light forces.
The aim of this study was to determine the optimal loading condition for pure intrusion of the six maxillary anterior teeth with miniscrews according to the amount of alveolar bone loss. For this purpose, three-dimensional (3D) finite element analysis was performed to investigate initial displacement and stress distribution in individual teeth according to various loading conditions with miniscrews and hooks, after setting the alveolar bone loss.
To calculate the tooth elements, the tooth morphologies were based on 3D scans of a dental model produced based on a sample survey of adults with normal occlusion in Japan (Model-i21D-400G; Nissin Dental Products, Kyoto, Japan). The positions and axial inclinations of the teeth were determined based on the ideal occlusion of Andrews, and the arch form was fabricated according to the broad arch form (Tru Arch®, Ormco, Glendora, CA, USA). The long axis of the incisor was inclined by 60° from the occlusal plane. The thickness of the periodontal ligament (PDL) was considered to be 0.25 mm, and this thickness was constant around the root surface.1213 The average alveolar bone thicknesses on the labial and lingual sides were determined from the 3D scans of the same dental model.
The morphology of the alveolar bone was modeled 1 mm above the cemento-enamel junction (CEJ) following the curvature of the CEJ in the case of normal bone level (i.e., bone loss = 0). Alveolar bone loss was assumed to be even in the bucco-lingual and antero-posterior directions. In the 2-mm and 4-mm loss models, the alveolar bone was 3 mm and 5 mm above the CEJ respectively. The brackets were made based on a Microarch® (Tomy Co., Tokyo, Japan), and the bracket slots were located on the facial axes of the clinical crowns of the individual teeth based on the Andrews plane. The arch wire was made from 0.017 × 0.025-inch stainless steel wire, and the brackets for all teeth were ligated firmly to the arch wire.
As previously described,14 a finite element model was created for a segment of the six maxillary anterior teeth in order that it could be interpreted as an independent model, that was not affected by the posterior teeth, and the boundary condition between the arch wire and the bracket was set to "no play". The teeth, alveolar bone, PDL, and brackets were all generated with 3D tetrahedral elements. As each tooth was an independent model, it was contacted with the adjacent teeth at the contact points and was connected with the arch wire through the bracket. In order to simulate free sliding of the arch wire, the friction resistance in the interface between the arch wire and the brackets was ignored.1516
The miniscrews were assumed to be located 12 mm gingivally to the arch wire at the midpoints between the adjacent roots of the teeth antero-posteriorly, and just below the arch wire bucco-lingually. The bracket-tooth, bracket-arch wire, and bone-miniscrew interfaces were defined as fully bonded surfaces. The x axis was set in the medial-lateral direction, the y axis was set in the anterior-posterior direction, and the z axis was set in the superior-inferior direction. A +x value was defined as the lateral direction, −y as the posterior direction, and +z as the superior direction, to analyze the displacement of the individual teeth under intrusive force (Figure 1).
All materials were assumed to be homogeneous, isotropic, and linear-elastic. The applied properties for each material are listed in Table 1. These properties were selected based on previous studies.1718 Although the Young's modulus and Poisson's ratio for the PDL are broad in range according to the literature, those utilized in the current study for the PDL followed those of previous studies on the intrusion or en-masse retraction of the segment of the six maxillary or mandibular anterior teeth using miniscrews.141920 Of course, this does not perfectly reflect the complex structure and behavior of the PDL. However, this assumption was sufficient to describe initial tooth displacement in the six-tooth anterior segment under orthodontic loading.
For each segment of six anterior teeth, the points and directions of the intrusive force were applied by varying the locations of the hooks and miniscrews (Table 2, Figure 2). The magnitude of the intrusive force applied to the four maxillary incisors was initially suggested to be approximately 100 g.21 On the other hand, Ricketts22 proposed a magnitude of 125–160 g. In the present study, for the single force of the inter-central incisors, 100 g was applied to the six-tooth anterior segment. In the bilateral force conditions, 50 g of intrusive force per side was applied to the six-tooth anterior segment.
The analyses were performed with ANSYS software (version 12.0; ANSYS Inc., Canonsburg, PA, USA). Using the finite element method, the initial displacement of the anterior teeth and the von Mises stresses along the root surface were evaluated. In order to measure the displacement of the tooth under the intrusive force, the nodes were selected to be at the midpoints of the incisal edges and root apices of individual teeth, and the movements of the nodes were considered to be tooth displacement. The displacements of the nodes that appeared in the three directions (i.e., the x, y, and z axes) were calculated by obtaining the coordinates. In order to measure the degree of labial tilting of the individual teeth according to bone loss, it was calculated via the angles between the longitudinal axes of each tooth before and after loading of the intrusive force, on the sagittal view.
Without bone loss, intrusion occurred in all of the central incisor, lateral incisor, and canine cases and was accompanied by labial tipping in six of the conditions in which the applied force directions were set differently (Figure 3, Table 3). In case A, the central incisor was labially inclined 32.9 millidegrees under the intrusive force. The midpoint of the incisor edge of the central incisor moved medially 3.9 µm, anteriorly 8.6 µm, and superiorly 5.7 µm (Table 4). The apex of the central incisor moved laterally 3.0 µm, posteriorly 4.2 µm, and superiorly 5.6 µm in case A. The amounts of medial-lateral, anterior-posterior, and vertical displacement of the central incisor tip and root apex were the largest in case A. The degree of uncontrolled tipping of the central incisor decreased in the order of cases B, C, D, E, and F. In case F, the midpoint of the incisor edge of the central incisor moved laterally 0.3 µm, anteriorly 1.2 µm, and superiorly 1.4 µm. The apex of the central incisor moved posteriorly 0.5 mm and superiorly 1.5 µm. The amount of anterior-posterior displacement of the central incisor was negligible, and the lowest vertical displacement of the central incisor tip and root apex was observed in case F.
With regard to the lateral incisor, the midpoint of the incisor edge of the lateral incisor moved medially 2.2 µm, anteriorly 2.9 µm, and superiorly 2.9 µm in case B (Table 4). Cases B, C, and D exhibited uncontrolled labial tipping with significant amounts of vertical displacement. In case D, the midpoint of the incisor edge of the canine moved medially 1.0 µm, anteriorly 2.7 µm, and superiorly 2.1 µm. The anterior-posterior and vertical displacements of the canine were the greatest in case D. In case A, the minimum amount of horizontal displacement of the canine was observed.
With 2 mm of bone loss, in all six conditions of varying applied force directions, the degree of labial tilting and the amounts of anterior-posterior and vertical displacement were larger than when there was no bone loss (Tables 3 and 4). Cases A, B, and C, all exhibited uncontrolled tipping of the central and lateral incisors (Figure 4). With 4 mm of bone loss, the overall pattern of tooth movement was similar to that of the 0- and 2-mm bone loss conditions, but the degree of labial tilting movement was more severe (Table 3, Figure 5). The case F showed relatively lower values in the degree of labial tilting and the amounts of medial-lateral and anterior-posterior displacements of the six anterior teeth than the other cases, which was similar in pattern with 0- and 2-mm bone loss conditions.
In all cases, more von Mises stress was applied to the apex than the cervical area of the PDL (Table 5).
In case A, the maximum von Mises stresses on the central incisor apical and cervical areas were 2.8 g/mm2 and 1.1 g/mm2 respectively. In case B, the stresses on the lateral incisor apical and cervical areas were 1.3 g/mm2 and 0.5 g/mm2 respectively. The stress on the central incisor was greatest in case A, and that on the lateral incisor was greatest in case B. The maximum von Mises stress on the canine was relatively lower than it was on other types of tooth. In case F, the maximum von Mises stresses were relatively evenly distributed across the central incisor, lateral incisor, and canine.
When bone loss was increased to 2 mm, the amount of stress increased further. In case A, the stress that was concentrated on the central incisor was noticeable, and with regard to the lateral incisor, case B exhibited the highest stress value. In case F, the stress was relatively evenly distributed. When bone loss was increased to 4 mm, in case A the maximum von Mises stresses on the central incisor apical and cervical areas were 3.9 g/mm2 and 1.2 g/mm2 respectively. In case B, the stresses on the lateral incisor apical and cervical areas were 2.0 g/mm2 and 0.7 g/mm2 respectively. The stress distribution pattern was the same as those observed at bone loss levels of 0 and 2 mm, but the stress was markedly increased (Figure 6).
When intrusive force was applied to the six maxillary anterior teeth with miniscrews and hooks in different positions, the central incisor, lateral incisor, and canine all underwent intrusion accompanied by labial tilting movement. The degree of labial tilting varied depending on the locations of the miniscrews and hooks, because force control involves not only force magnitude but also the points of force application and direction (line of action).23 The movement of a segment can be determined by the net effect of all forces on it, thus it is necessary to combine applied forces to determine a resultant force.23 In case A, the central incisor exhibited pseudo-intrusion due to a significant amount of labial tilting of the tooth (Table 3). As the horizontal force component (a retraction force) increased and the vertical intrusive force component decreased, the amount of anterior-posterior displacement of the central incisor gradually decreased in the order of cases A, B, C, E, D, and F (Table 4). In the lateral incisor, cases B, C, and D exhibited the greatest amounts of labial tilting. In the canine, case D exhibited a noticeably greater amount of labial tilting compared with the other conditions, because at the distal part of the canine, a retraction force was not added as only a vertical force was applied onto the arch. In case F, the hook location was mesial to the canine and a considerable amount of labial tilting occurred, but this amount was smaller than that observed in case D.
Therefore, within the limitations of this study, minimal labial displacement of the six anterior teeth was observed when the hook was positioned distal to the lateral incisor, and the miniscrews were inserted between the canine and the first premolar (case F). In this condition, relatively pure intrusion was observed. This pattern was likely observed because the force was applied closest to the CR among the six anterior teeth.24 In other words, these findings imply that the intrusion method involving the miniscrew on the posterior side of the canine and the hook on the distal side of the lateral incisor is effective for achieving relatively pure intrusion of the six anterior teeth. This result is encouraging, because treatment will be much more effective if the six anterior teeth can be simultaneously intruded by a predictable method. However, in order to intrude only a single tooth such as the central or lateral incisor, the application of this method may not be effective. Particularly when the maxillary anterior teeth are flared and extruded due to periodontal problems, intrusive movements should be attempted with bodily movements or slight linguoversion. In the view of the authors of this study, additional retraction horizontal force is necessary in addition to intrusion force passing by the CR, to achieve pure intrusion of the six maxillary anterior teeth.
When the same force was applied with the six anterior teeth being intruded as a segment, the degree of labial tilting of the anterior teeth increased in all cases as bone loss increased (Table 3). Moreover, the difference in tooth displacement was greater when bone loss was increased from 2 to 4 mm than when it was increased from 0 to 2 mm in the anterior tooth segment (Table 4). Furthermore, the difference in labial tilting was much larger than the difference in intrusion.
A number of studies have investigated the location of the CR of maxillary anterior teeth.1825 With regard to the horizontal location of the CR of the anterior teeth, it has been reported that the CR moves posteriorly as alveolar bone loss occurs, and that the posterior movement of the CR increases as alveolar bone loss increases.26 It is likely that because the posterior movement of the CR was greater as bone loss was increased from 2 to 4 mm than when it was increased from 0 to 2 mm, the anterior-posterior displacement of teeth would be greater going from 2 to 4 mm under the same force vector. This result was likely caused by a decrease in the alveolar bone support of the anterior part due to the reduction in alveolar bone, because the teeth and alveolar bone of the anterior part were tilted anteriorly.
More von Mises stress was concentrated on the tooth apex area than on the cervical area of the PDL in all cases. As the displacement of the tooth became larger, the maximum von Mises stress also exhibited a greater tendency to increase (Table 5); as in case A when the movement of the central incisor was sufficiently large, the maximum von Mises stress was also concentrated on the central incisor rather than the other teeth. The stress level was lowest, and the stress was evenly distributed when the miniscrew was installed on the distal part of the canine, and the intrusion force was applied on the distal part of the lateral incisor. When there is bone loss, the PDL naturally diminishes, so smaller PDL surfaces should be able to withstand the stress caused by tooth movement. Therefore, it is physiologically desirable that lower levels of stress be distributed evenly along the PDL, to prevent external apical root resorption.2728
The design of this study has some limitations. The study intended to mathematically visualize the initial displacement and stress distribution of the PDL during intrusion using finite element analysis; thus, the results may not exactly reflect clinical outcomes, which are influenced by the cumulative effects of continuous bone reactions and rebounding of the arch wire due to secondary displacement of the teeth. In other words, the prediction of ultimate clinical outcomes based on arithmetic calculations of the initial response should be avoided. Additionally, due to technical difficulties, it did not measure the change in the location of the CR of individual teeth according to the amount of alveolar bone loss. If the change in the location of the CR was quantitatively analyzed, the effect of bone loss on the tooth displacement pattern under intrusive force could be more clearly described. Additional limitations of this study include the constant values that were used for the physical properties of the tissues (these values would normally vary clinically due to the histologic process), and the assumptions that the periodontal membrane was homogeneous, isotropic, and uniform in thickness. These limitations could result in differences between clinical applications and simulation studies. Moreover, due to individual variation, it is impossible to create a mathematical model that exactly simulates each case. Recently, the use of 3D computed tomography (CT) has increased, and this method could be used to create individual tooth models that would enable simulations of the orthodontic tooth movements of each patient. Furthermore, the combination of 3D CT-assisted individual models with finite element analysis software would aid clinical treatment planning.
When the same force was applied with the six maxillary anterior teeth being intruded as a segment, the degree of labial tilting of the anterior teeth increased in all cases as bone loss increased. It is likely possible to induce initial tooth displacement that is similar to pure intrusion when miniscrews are inserted distal to the maxillary canines and intrusion forces are applied distal to the lateral incisors. In this condition, the maximum von Mises stress is relatively evenly distributed across the central incisor, lateral incisor, and canine regardless of the amount of alveolar bone loss.
Figures and Tables
 | Figure 1Three-dimensional finite element models and the coordination system. A, Model of the maxillary teeth. B, C, and D, Lateral views of the models with 0, 2, and 4 mm of alveolar bone loss respectively. A +x value was defined as the lateral direction, −y as the posterior direction, and +z as the superior direction, to analyze the displacement of individual teeth. |
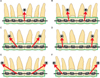 | Figure 2Force vectors for the intrusion of the six-tooth anterior segment (green box). Black and white circles, miniscrew heads; red arrows, load vectors. |
 | Figure 3Sagittal view of the displacement of the anterior teeth without bone loss in the six-tooth anterior segment. Each line represents the longitudinal axis of each tooth. The displacement is scaled up 1,000-fold to enhance visibility. Each figure refers to the each loading condition (See also Figure 2). Dotted line, before the displacement of the individual teeth; solid line, after the displacement of the individual teeth. |
 | Figure 4Sagittal view of the displacement of the anterior teeth with 2 mm of bone loss in the six-tooth anterior segment. Each line represents the longitudinal axis of each tooth. The displacement is scaled up 1,000-fold to enhance visibility. Each figure refers to the each loading condition (See also Figure 2). Dotted line, before the displacement of the individual teeth; solid line, after the displacement of the individual teeth. |
 | Figure 5Sagittal view of the displacement of the anterior teeth with 4 mm of bone loss in the six-tooth anterior segment. Each line represents the longitudinal axis of each tooth. The displacement is scaled up 1,000-fold to enhance visibility. Each figure refers to the each loading condition (See also Figure 2). Dotted line, before the displacement of the individual teeth; solid line, after the displacement of the individual teeth. |
 | Figure 6Comparison of the maximum compressive stress distributions in periodontal ligaments with 4 mm of bone loss. Blue color indicates high-stress distribution, and red color indicates low-stress distribution. Each figure refers to the each loading condition (See also Figure 2). |
Table 3
The degree of labial tilting of individual teeth before and after loading of the intrusive force according to bone loss (millidegrees)

References
1. Kang DY, Choi SH, Jung YS, Hwang CJ. Interdisciplinary treatment for an adult patient with anterior open bite, severe periodontitis, and intellectual disability. J Craniofac Surg. 2015; 26:e240–e244.

2. Serio FG, Hawley CE. Periodontal trauma and mobility. Diagnosis and treatment planning. Dent Clin North Am. 1999; 43:37–44.
3. Rohatgi S, Narula SC, Sharma RK, Tewari S, Bansal P. A study on clinical attachment loss and gingival inflammation as etiologic factors in pathologic tooth migration. Niger J Clin Pract. 2011; 14:449–453.

4. Towfighi PP, Brunsvold MA, Storey AT, Arnold RM, Willman DE, McMahan CA. Pathologic migration of anterior teeth in patients with moderate to severe periodontitis. J Periodontol. 1997; 68:967–972.

5. Kanomi R. Mini-implant for orthodontic anchorage. J Clin Orthod. 1997; 31:763–767.
6. Nishimura M, Sannohe M, Nagasaka H, Igarashi K, Sugawara J. Nonextraction treatment with temporary skeletal anchorage devices to correct a Class II Division 2 malocclusion with excessive gingival display. Am J Orthod Dentofacial Orthop. 2014; 145:85–94.

7. Choi JH, Yu HS, Lee KJ, Park YC. Three-dimensional evaluation of maxillary anterior alveolar bone for optimal placement of miniscrew implants. Korean J Orthod. 2014; 44:54–61.

8. Lee KJ, Park YC, Hwang CJ, Kim YJ, Choi TH, Yoo HM, et al. Displacement pattern of the maxillary arch depending on miniscrew position in sliding mechanics. Am J Orthod Dentofacial Orthop. 2011; 140:224–232.

9. Cobo J, Argüelles J, Puente M, Vijande M. Dentoalveolar stress from bodily tooth movement at different levels of bone loss. Am J Orthod Dentofacial Orthop. 1996; 110:256–262.

10. Cobo J, Sicilia A, Argüelles J, Suárez D, Vijande M. Initial stress induced in periodontal tissue with diverse degrees of bone loss by an orthodontic force: tridimensional analysis by means of the finite element method. Am J Orthod Dentofacial Orthop. 1993; 104:448–454.

11. Cattaneo PM, Dalstra M, Melsen B. The finite element method: a tool to study orthodontic tooth movement. J Dent Res. 2005; 84:428–433.

12. Coolidge ED. The thickness of the human periodontal membrane. J Am Dent Assoc. 1937; 24:1260–1270.

13. Kronfeld R. Histologic study of the influence of function on the human periodontal membrane. J Am Dent Assoc. 1931; 18:1242–1274.

14. Park HK, Sung EH, Cho YS, Mo SS, Chun YS, Lee KJ. 3-D FEA on the intrusion of mandibular anterior segment using orthodontic miniscrews. Korean J Orthod. 2011; 41:384–398.

15. Sung EH, Kim SJ, Chun YS, Park YC, Yu HS, Lee KJ. Distalization pattern of whole maxillary dentition according to force application points. Korean J Orthod. 2015; 45:20–28.

16. Sung SJ, Baik HS, Moon YS, Yu HS, Cho YS. A comparative evaluation of different compensating curves in the lingual and labial techniques using 3D FEM. Am J Orthod Dentofacial Orthop. 2003; 123:441–450.

17. Vollmer D, Bourauel C, Maier K, Jäger A. Determination of the centre of resistance in an upper human canine and idealized tooth model. Eur J Orthod. 1999; 21:633–648.

18. Reimann S, Keilig L, Jäger A, Bourauel C. Biomechanical finite-element investigation of the position of the centre of resistance of the upper incisors. Eur J Orthod. 2007; 29:219–224.

19. Lee EH, Yu HS, Lee KJ, Park YC. Three dimensional finite element analysis of continuous and segmented arches with use of orthodontic miniscrews. Korean J Orthod. 2011; 41:237–254.

20. Mo SS, Kim SH, Sung SJ, Chung KR, Chun YS, Kook YA, et al. Torque control during lingual anterior retraction without posterior appliances. Korean J Orthod. 2013; 43:3–14.

22. Ricketts RM. Bioprogressive therapy as an answer to orthodontic needs. Part I. Am J Orthod. 1976; 70:241–268.

24. Polat-Ozsoy O, Arman-Ozcirpici A, Veziroglu F. Miniscrews for upper incisor intrusion. Eur J Orthod. 2009; 31:412–416.

25. Sia S, Koga Y, Yoshida N. Determining the center of resistance of maxillary anterior teeth subjected to retraction forces in sliding mechanics. An in vivo study. Angle Orthod. 2007; 77:999–1003.

26. Sung SJ, Kim IT, Kook YA, Chun YS, Kim SH, Mo SS. Finite-element analysis of the shift in center of resistance of the maxillary dentition in relation to alveolar bone loss. Korean J Orthod. 2009; 39:278–288.





 PDF
PDF ePub
ePub Citation
Citation Print
Print





 XML Download
XML Download