Abstract
Objective
The aim of this study was to investigate whether labial tooth inclination and alveolar bone loss affect the moment per unit of force (Mt/F) in controlled tipping and consequent stresses on the periodontal ligament (PDL).
Methods
Three-dimensional models (n = 20) of maxillary central incisors were created with different labial inclinations (5°, 10°, 15°, and 20°) and different amounts of alveolar bone loss (0, 2, 4, and 6 mm). The Mt/F necessary for controlled tipping (Mt/Fcont) and the principal stresses on the PDL were calculated for each model separately in a finite element analysis.
Results
As labial inclination increased, Mt/Fcont and the length of the moment arm decreased. In contrast, increased alveolar bone loss caused increases in Mt/Fcont and the length of the moment arm. When Mt/F was near Mt/Fcont, increases in Mt/F caused compressive stresses to move from a predominantly labial apical region to a palatal apical position, and tensile stresses in the labial area moved from a cervical position to a mid-root position. Although controlled tipping was applied to the incisors, increases in alveolar bone loss and labial tooth inclination caused increases in maximum compressive and tensile stresses at the root apices.
With increasing numbers of adult patients seeking orthodontic care, it is becoming more common to treat patients with periodontal disease. Common characteristics of dentition with periodontal disease include labial flaring of the maxillary anterior teeth, diastema, irregular interdental spacing, rotation, extrusion, tipping, and drifting.1 A recent study showed that labial flaring due to pathologic migration of the maxillary anterior teeth was a common complication of moderate-to-severe periodontitis and often motivated patients to seek periodontal and orthodontic therapy.2
Several studies have investigated teeth in the upright position to evaluate stress distributions and movement patterns in teeth with different amounts of alveolar bone loss.3456 However, the maxillary incisors are typically labially inclined by about 60° toward the occlusal plane rather than positioned upright.7 Moreover, the incisors are often more labially inclined when pathologic tooth migration is present in patients with alveolar bone loss28; therefore, the force vector is rarely perpendicular to the long axis of the incisor.
Often, to correct a flared incisor in a patient with horizontal bone loss, the minimum moment per unit force (controlled tipping) is delivered with the expectation that the lowest stress is applied to the root apex.9 However, when orthodontic force is applied to a tooth with alveolar bone loss, the strain-stress distribution changes in the periodontal ligament (PDL).10 This is important, because the magnitude and direction of an orthodontic load determines whether the tissue reacts with bone formation, bone resorption, or iatrogenic external apical root resorption.1112 Recent studies that used three-dimensional (3D) imaging technology suggested that a large force or high stress on the PDL led to external apical root resorption.131415
The aim of this study was to investigate how tooth inclination and bone loss affected the amount of moment per unit of force (Mt/F) needed for controlled tipping and consequent stresses on the PDL. Our null hypothesis was that the stress on the PDL imposed by controlled tipping applied to the incisor would not change with different tooth inclinations or with different amounts of alveolar bone loss.
Stress on the PDL and tooth movements were investigated with a finite element analysis program (ANSYS ver. 12.1; Swanson Analysis System, Canonsburg, PA, USA). We created 3D models (n = 20) of maxillary central incisors with different labial inclinations and different amounts of alveolar bone loss (Figure 1). Tooth morphology was based on 3D scans of dental models produced from a sample survey of adults with normal occlusion in Japan (Model-i21D-400G; Nissin Dental Products, Kyoto, Japan). An orthodontic bracket was modeled based on the Micro-arch® (Tomy Co., Tokyo, Japan) structure. The PDL was 0.25 mm thick, and formed an even layer over the entire root surface.1617 The alveolar bone was modeled 1 mm above the cementoenamel junction (CEJ), and followed the curvature of the CEJ in cases with normal bone levels. The axis of the normal incisor was inclined 60° from the occlusal plane.7
The long axes of the abnormal incisor models were inclined facially at 0°, 5°, 10°, 15°, and 20° relative to the axis of the normal incisor model (Figure 2). Alveolar bone loss (0, 2, 4, and 6 mm) was assumed to be equivalent in the buccolingual and mesiodistal directions. As a result, in a model with 2 mm of bone loss, alveolar bone extended to 3 mm above the CEJ, and so on. Each of the 20 models consisted of an incisor, a PDL, and alveolar bone. All structures were subdivided into elements and nodes with a 3D brick tetrahedral mesh.
Each of the 20 models was subjected to 3 loads applied to the crown as follows.
1. A retraction force (F) of 100 gf that caused the crown to move lingually.
2. A counter-tipping moment of force (Mt) that caused the crown to move in the labial direction. The Mt was created by applying coupled forces. The length of the moment arm was 1.5 mm. The Mt/F ratio varied from 0 to 10 (Figure 3A).
3. A counter-rotation moment (Mr) resulted in a distolingual rotation designed to counteract the confounding moment generated by the asymmetric morphology of the incisor (Figure 3B).20 The Mr was created by applying a coupled force, and calculated such that the mesial and distal ends of the incisal edge moved equal distances in the same direction. The length of the moment arm was 3.6 mm. Because the retraction force and length of the moment arm were the same regardless of the experimental conditions, Mr was set to be constant to prevent undesirable tooth rotation under all conditions. For the boundary condition, all nodes at the base of the model (bone) were fixed in all directions to constrain free-body motion.
Controlled tipping (Mt/Fcont) was defined as the force that caused minimum movement of the root apex. The total displacement of the apical root node was the sum of the displacements in 3 dimensions, as shown in Figure 3C. According to the definition given by Burstone,9 the center of rotation should be at the apex when controlled tipping is simulated. A simple, two-dimensional conceptualization of that definition is that the Δy (anterior-posterior displacement) at the apex would be 0 with controlled tipping. In this study, even though rotation was controlled with a counter-rotational moment (Mr), the direction of the force caused an intrusion effect on the teeth due to the 60° inclination. As a result, the apex moved both two-dimensionally (anterior-posterior) and three-dimensionally. We defined the 3D movement (Δ) as follows:
Thus, controlled tipping was defined as the smallest possible Δ for a given bone level and tooth inclination. Because of the intrusive movement of the apex, the smallest Δ was always > 0 (Figure 3). The amount of moment per unit of force (Mt/F ratio) necessary for controlled tipping (Mt/Fcont) was calculated for each model separately.
Stress on the PDL was calculated in terms of principal stresses. The principal stresses were identified by determining the planes at which the traction vector was purely normal with no shear component. In a 3D stress field, there are 3 principal stress components ranked in descending order. Among these principal stresses on the PDL, the maximum value of the first principal stress (S1, maximum tensile stress) and the minimum value of the third principal stress (S3, maximum compressive stress) were recorded for each model and each M/F ratio.
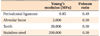
For each amount of labial inclination and alveolar bone loss of the incisors, we investigated Mt/Fcont ratios for controlled tipping. We found that as labial inclination increased, Mt/Fcont decreased. In contrast, increases in alveolar bone loss caused increases in Mt/Fcont (Table 2). For the incisor with 6 mm of bone loss combined with an inclination of 20°, the Mt/Fcont was 6.5, which was lower than that of the normal tooth (Mt/Fcont, 8.5).
Additionally, Figure 4 shows that as labial inclination increased, the length of the moment arm (the perpendicular distance between the line of action of the force and the center of resistance) decreased. In contrast, an increase in alveolar bone loss caused increases in the length of the moment arm. The position of the center of resistance associated with alveolar bone loss conditions was defined in reference to a previous study.5
For each inclination and simulated bone loss amount, we plotted the relationship between the Mt/F ratio and principal stresses on the PDL (Figure 5). As bone loss increased, a given Mt/F imparted greater maximum tensile and compressive stresses on the PDL. Moreover, in teeth with greater alveolar bone loss, the stress changed more abruptly with changes in the Mt/F ratio. When the labial inclination of the incisor was increased without changing the amount of alveolar bone loss, the Mt/F ratio decreased for the lowest principal stress.
The patterns of stress distributed over the root were examined under conditions with an Mt/F value near that of Mt/Fcont (Figure 6). With increases in the Mt/F ratio near Mt/Fcont, compressive stresses dominant in the labial apical region moved to the palatal apical area (Mt/F above Mt/Fcont). In contrast, tensile stresses dominant in the labial area (Mt/F below Mt/Fcont) moved from the cervical to the mid-root regions (Mt/F above Mt/Fcont). These patterns were similar under all experimental conditions.
Previous studies analyzed upright incisors and forces applied perpendicular to the occlusal plane.342122 Moreover, many studies that investigated alveolar bone loss and stress distributions did not consider the facial inclination of teeth.34523 The present study evaluated how tooth inclination and bone loss affected the M/F ratio in controlled tipping and the resulting PDL stresses. We chose the maxillary central incisor because it typically undergoes the most detailed tooth movement, and carries a higher risk of external apical root resorption than all other teeth, except for the maxillary lateral incisor.24
Often, correction of a flared incisor with alveolar bone loss involves a combination of retraction and intrusion. A mere retraction of flared teeth would lead to deepening of the bite.25 In the present study, force was applied parallel to the occlusal plane without an intrusive vector. Adding an intrusive force would incline the direction of the net force; this result would be comparable to applying a horizontal force to a tooth with a greater inclination. Therefore, we did not apply an intrusive force in this study (Figure 3).
Geramy5 reported that alveolar bone loss caused the center of resistance to move toward the apex; however, its relative distance to the alveolar crest decreased at the same time. Figure 4 also shows that alveolar bone loss caused increases in the length of the moment arm. Siatkowski26 reported that an increase of 38% was needed to obtain bodily movement when 5 mm of alveolar bone loss had occurred. Therefore, the applied force and moment magnitudes must be reduced proportionally to maintain physiologically tolerable movement, because reduced support of the PDL and alveolar bone causes changes in the center of resistance.52728
The incisor was modeled at inclinations over an average range (0° to 20°), both with and without different levels of alveolar bone loss (0 to 6 mm). When controlled tipping was applied to the incisor, the maximum compressive stress migrated along the y-axis to the root apex, and the maximum tensile stress migrated from the cervical area to the middle of the root. This distribution of stress was consistent with the findings of Kanjanaouthai et al.20 They found that incisors with a high degree of inclination experienced more compressive stress concentrated at the apices than incisors that were more upright. Labial inclination resulted in an increased intrusive force component directed parallel to the long axis; that force enhanced compressive stress at the apex and tension in the PDL in the longitudinal direction (Figure 6).
As a result, facial inclination of the incisor caused a concentration of compressive stress at the apex; thus, controlled tipping of an incisor with a corrective force may lead to increased root resorption.24 This interpretation is consistent with findings reported in the literature, which suggested that increases in the angle between the central incisor and palatal plane were strongly correlated with increases in external apical root resorption.29 Furthermore, it was shown that the risk of external apical root resorption was enhanced by the movements of teeth with mature roots, extensive movement of the root, and intrusive mechanics.30
Previous studies showed that teeth with alveolar bone resorption experienced increased maximum tensile and compressive stresses relative to those found in teeth with normal bone heights.6 In the present study, increasing the bone loss in teeth without changing the incisal inclination caused increased root apex displacements and greater maximum compressive and tensile stresses. However, for each level of alveolar bone loss, these stresses could be reduced with controlled tipping versus uncontrolled tipping or root movements. This suggested that in patients with alveolar bone loss, an inadequate force system might cause radical root apex displacement and stress. External root resorption occurs when forces at the apex exceed the resistance and reparative ability of periapical tissues29; thus, applied force and moment magnitudes must be reduced proportionately to maintain physiologically tolerable movements without causing further damage to supporting structures.5
The present study had some limitations. First, we used principal stresses to evaluate stress distributions in the PDL. For the PDL, the 3 principal stresses are very approximate because of the low values of Young's modulus, and because Poisson's ratio approached 0.5, which represents that of water. Thus, at a certain node in the PDL, the 3 principal stresses were either compressive or tensile, with values approximating those found in nature. Second, the PDL was not constructed with a thickness that varied from the cervix to apex. In addition, alveolar bone loss was assumed to occur only in the horizontal direction, and the amounts were equivalent in the buccolingual and mesiodistal directions.
When controlled tipping was applied to incisors, alveolar bone loss and labial tooth inclination caused increases in the maximum compressive and tensile stresses at the root apices. This finding suggested that an inadequate force system applied to facially inclined incisors might cause greater stress at the root apices and induce external apical root resorption in teeth with alveolar bone loss compared with teeth without bone loss.
Figures and Tables
Figure 1
The basic model of an incisor. A, Tooth length, 24.2 mm; tooth root, 13.2 mm; bracket position midpoint, 4.5 mm. The alveolar bone (dark blue) is situated 1 mm above the cementoenamel junction (CEJ). B, Normal inclination of an incisor 60° from the occlusal plane. C, A tetrahedral mesh covers the tooth surface and bracket.
M, Mesial; D, distal; B, buccal; P, palatal.

Figure 2
Models of incisors with different inclinations and different amounts of alveolar bone loss. A total of 20 models were created (5 different inclinations and 4 levels of bone loss). A, Experimental incisor models with labial inclinations of 5°, 10°, 15°, and 20°. B, Models demonstrating 0, 2, 4, and 6 mm of alveolar bone loss.

Figure 3
Forces associated with the 3 different loads applied to the tooth models. A, The first load (1) was a retraction force (100 gf) applied parallel to the occlusal plane at the midpoint of the bracket. The second load was a coupled force (2, 3) that created a counter-tipping moment (Mt). B, The third load was a coupled force (4, 5) that created a rotation moment (Mr). C, Calculation of the three-dimensional displacement (Δ) of the apex.

Figure 4
Changes in the length of the moment arm (the perpendicular distance between the line of action of the force and the center of resistance) according to changes in maxillary central incisor inclination and surrounding alveolar bone loss.

Figure 5
Relationship between the Mt/F ratio and principal stresses in tooth models with 20° of labial inclination. The minimum principal stress levels are indicated by triangles.

Figure 6
Incisor root stress distribution patterns under conditions with Mt/F near Mt/Fcont (red area, maximum tensile stress; blue area, maximum compressive stress). A, A tooth model with no alveolar bone loss and an incisal inclination of 15°; controlled tipping occurs at Mt/F = 6. B, A tooth model with 6 mm of alveolar bone loss and an incisal inclination of 15°; controlled tipping occurs at Mt/F = 8.

References
1. Gkantidis N, Christou P, Topouzelis N. The orthodontic-periodontic interrelationship in integrated treatment challenges: a systematic review. J Oral Rehabil. 2010; 37:377–390.

2. Towfighi PP, Brunsvold MA, Storey AT, Arnold RM, Willman DE, McMahan CA. Pathologic migration of anterior teeth in patients with moderate to severe periodontitis. J Periodontol. 1997; 68:967–972.

3. Cobo J, Argüelles J, Puente M, Vijande M. Dentoalveolar stress from bodily tooth movement at different levels of bone loss. Am J Orthod Dentofacial Orthop. 1996; 110:256–262.

4. Cobo J, Sicilia A, Argüelles J, Suárez D, Vijande M. Initial stress induced in periodontal tissue with diverse degrees of bone loss by an orthodontic force: tridimensional analysis by means of the finite element method. Am J Orthod Dentofacial Orthop. 1993; 104:448–454.

5. Geramy A. Alveolar bone resorption and the center of resistance modification (3-D analysis by means of the finite element method). Am J Orthod Dentofacial Orthop. 2000; 117:399–405.

6. Geramy A. Initial stress produced in the periodontal membrane by orthodontic loads in the presence of varying loss of alveolar bone: a three-dimensional finite element analysis. Eur J Orthod. 2002; 24:21–33.

7. McLaughlin RP, Bennett JC, Trevisi HJ. Systemized orthodontic treatment mechanics. Edinburgh: Mosby;2001.
8. Tian YL, Liu F, Sun HJ, Lv P, Cao YM, Yu M, et al. Alveolar bone thickness around maxillary central incisors of different inclination assessed with cone-beam computed tomography. Korean J Orthod. 2015; 45:245–252.

9. Burstone CJ. The mechanics of the segmented arch techniques. Angle Orthod. 1966; 36:99–120.
10. Cattaneo PM, Dalstra M, Melsen B. Moment-to-force ratio, center of rotation, and force level: a finite element study predicting their interdependency for simulated orthodontic loading regimens. Am J Orthod Dentofacial Orthop. 2008; 133:681–689.

11. Brezniak N, Wasserstein A. Orthodontically induced inflammatory root resorption. Part II: The clinical aspects. Angle Orthod. 2002; 72:180–184.
12. Brezniak N, Wasserstein A. Orthodontically induced inflammatory root resorption. Part I: The basic science aspects. Angle Orthod. 2002; 72:175–179.
13. Viecilli R, Katona T, Roberts W. Optimization of microCT data processing for modelling of dental structures in orthodontic studies. Comput Methods Biomech Biomed Engin. 2007; 10:257–263.

14. Viecilli RF, Katona TR, Chen J, Hartsfield JK Jr, Roberts WE. Three-dimensional mechanical environment of orthodontic tooth movement and root resorption. Am J Orthod Dentofacial Orthop. 2008; 133:791.e11–791.e26.

15. Cheng LL, Türk T, Elekdağ-Türk S, Jones AS, Petocz P, Darendeliler MA. Physical properties of root cementum: Part 13. Repair of root resorption 4 and 8 weeks after the application of continuous light and heavy forces for 4 weeks: a microcomputed-tomography study. Am J Orthod Dentofacial Orthop. 2009; 136:320.e1–320.e10. discussion 320-1.

16. Richardson ER. Comparative thickness of the human periodontal membrane of functioning versus non-functioning teeth. J Oral Med. 1967; 22:120–126.
17. Coolidge ED. The thickness of human periodontal membrane. J Am Dent Assoc. 1937; 24:1260–1270.
18. Mo SS, Kim SH, Sung SJ, Chung KR, Chun YS, Kook YA, et al. Torque control during lingual anterior retraction without posterior appliances. Korean J Orthod. 2013; 43:3–14.

19. Sung EH, Kim SJ, Chun YS, Park YC, Yu HS, Lee KJ. Distalization pattern of whole maxillary dentition according to force application points. Korean J Orthod. 2015; 45:20–28.

20. Kanjanaouthai A, Mahatumarat K, Techalertpaisarn P, Versluis A. Effect of the inclination of a maxillary central incisor on periodontal stress: finite element analysis. Angle Orthod. 2012; 82:812–819.

21. Tanne K, Sakuda M, Burstone CJ. Three-dimensional finite element analysis for stress in the periodontal tissue by orthodontic forces. Am J Orthod Dentofacial Orthop. 1987; 92:499–505.

22. Shaw AM, Sameshima GT, Vu HV. Mechanical stress generated by orthodontic forces on apical root cementum: a finite element model. Orthod Craniofac Res. 2004; 7:98–107.

24. Sameshima GT, Sinclair PM. Predicting and preventing root resorption: Part I. Diagnostic factors. Am J Orthod Dentofacial Orthop. 2001; 119:505–510.

25. Melsen B. Adult orthodontics. Chichester, West Sussex: Blackwell Publishing;2012. p. 212.
26. Siatkowski RE. Optimal orthodontic space closure in adult patients. Dent Clin North Am. 1996; 40:837–873.
27. Tanne K, Nagataki T, Inoue Y, Sakuda M, Burstone CJ. Patterns of initial tooth displacements associated with various root lengths and alveolar bone heights. Am J Orthod Dentofacial Orthop. 1991; 100:66–71.

28. Sung SJ, Kim IT, Kook YA, Chun YS, Kim SH, Mo SS. Finite-element analysis of the shift in center of resistance of the maxillary dentition in relation to alveolar bone loss. Korean J Orthod. 2009; 39:278–288.





 PDF
PDF ePub
ePub Citation
Citation Print
Print


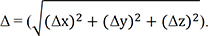
 XML Download
XML Download