1. Esposito M, Grusovin MG, Maghaireh H, Worthington HV. Interventions for replacing missing teeth: different times for loading dental implants. Cochrane Database Syst Rev. 2013; 3:CD003878.
2. Szmukler-Moncler S, Salama H, Reingewirtz Y, Dubruille JH. Timing of loading and effect of micromotion on bone-dental implant interface: review of experimental literature. J Biomed Mater Res. 1998; 43:192–203.
3. Misch CE, Wang HL, Misch CM, Sharawy M, Lemons J, Judy KW. Rationale for the application of immediate load in implant dentistry: Part I. Implant Dent. 2004; 13:207–217.
4. Brunski JB. Avoid pitfalls of overloading and micromotion of intraosseous implants. Dent Implantol Update. 1993; 4:77–81.
5. Kawahara H, Kawahara D, Hayakawa M, Tamai Y, Kuremoto T, Matsuda S. Osseointegration under immediate loading: biomechanical stress-strain and bone formation-resorption. Implant Dent. 2003; 12:61–68.
6. Chang PK, Chen YC, Huang CC, Lu WH, Chen YC, Tsai HH. Distribution of micromotion in implants and alveolar bone with different thread profiles in immediate loading: a finite element study. Int J Oral Maxillofac Implants. 2012; 27:e96–e101.
7. Javed F, Romanos GE. The role of primary stability for successful immediate loading of dental implants. A literature review. J Dent. 2010; 38:612–620.
8. Trisi P, Perfetti G, Baldoni E, Berardi D, Colagiovanni M, Scogna G. Implant micromotion is related to peak insertion torque and bone density. Clin Oral Implants Res. 2009; 20:467–471.
9. Wazen RM, Currey JA, Guo H, Brunski JB, Helms JA, Nanci A. Micromotion-induced strain fields influence early stages of repair at bone-implant interfaces. Acta Biomater. 2013; 9:6663–6674.
10. Winter W, Klein D, Karl M. Effect of model parameters on finite element analysis of micromotions in implant dentistry. J Oral Implantol. 2013; 39:23–29.
11. Mericske-Stern R, Assal P, Mericske E, Bürgin W. Occlusal force and oral tactile sensibility measured in partially edentulous patients with ITI implants. Int J Oral Maxillofac Implants. 1995; 10:345–353.
12. Waltimo A, Könönen M. Maximal bite force and its association with signs and symptoms of craniomandibular disorders in young Finnish non-patients. Acta Odontol Scand. 1995; 53:254–258.
13. Flanagan D, Ilies H, Lasko B, Stack J. Force and movement of non-osseointegrated implants: an in vitro study. J Oral Implantol. 2009; 35:270–276.
14. Fazel A, Aalai S, Rismanchian M. Effect of macro-design of immediately loaded implants on micromotion and stress distribution in surrounding bone using finite element analysis. Implant Dent. 2009; 18:345–352.
15. Freitas AC Jr, Bonfante EA, Giro G, Janal MN, Coelho PG. The effect of implant design on insertion torque and immediate micromotion. Clin Oral Implants Res. 2012; 23:113–118.
16. Engelke W, Decco OA, Rau MJ, Massoni MC, Schwarzwäller W. In vitro evaluation of horizontal implant micromovement in bone specimen with contact endoscopy. Implant Dent. 2004; 13:88–94.
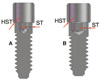
17. Steinebrunner L, Wolfart S, Ludwig K, Kern M. Implant-abutment interface design affects fatigue and fracture strength of implants. Clin Oral Implants Res. 2008; 19:1276–1284.









 PDF
PDF ePub
ePub Citation
Citation Print
Print



 XML Download
XML Download