PDF
ePub
Citation
Print
Abstract
Purpose
We measured angle lambda in normal and exotropic patients using Pentacam HR (Oculus, Wetzler, Germany). The relationship between angle lambda and refractive error was analyzed in the present study.
Methods
From December, 2013 to August, 2014, 135 eyes of 135 individuals under 16 years of age were enrolled in this study. Pentacam measures a distance between the center of the pupil and the corneal reflex point. The distance was converted to the angle lambda using the second law of cosines. All statistical analyses were performed using SPSS for Windows, version 21.0 (SPSS Inc, Chicago, IL, USA). The differences among the groups when measuring angle lambda were calculated using in-dependent t-test, Mann-Whitney test and analysis of variance (ANOVA). Pearson's correlation test was used to analyze the cor-relation among the variables.
Results
The mean age was 7.60 ± 3.68 years. Mean angle lambda values of the normal and exotropia groups were 4.41 ± 2.23° and 4.92 ± 2.58°, respectively. To evaluate the association of angle lambda and refraction measures, individuals were further classified according to the degree of myopia and hyperopia. The angle lambda values in the 3 groups according to refraction sta-tus (myopic, emmetropic and hyperopic) were 3.95 ± 2.16°, 4.84 ± 2.62° and 5.30 ± 2.35°, respectively. A positive correlation was observed between angle lambda and spherical equivalent (correlation coefficient = 0.341, p < 0.001). A significant negative correlation was observed between angle lambda and age (correlation coefficient = -0.181, p = 0.036). There was a negative cor-relation between axial length and angle lambda (correlation coefficient = -0.469, p = 0.003).
Conclusions
The results in this study were in agreement with the results from previous studies. Measuring the distance between the centers of the pupil and the point of corneal reflection using Pentacam is recommended as a quick, objective, quantitative and reproducible method to measure the angle lambda in children.
References
1. Basmak H, Sahin A, Yildirim N. . Measurement of angle kappa with synoptophore and Orbscan II in a normal population. J Refract Surg. 2007; 23:456–60.
2. Uozato H, Guyton DL. Centering corneal surgical procedures. Am J Ophthalmol. 1987; 103:264–75.
3. Braaf B, van de Watering TC, Spruijt K. . Calculating angle lambda (λ) using zernike tilt measurements in specular reflection corneal topography. Journal of Optometry. 2009; 2:207–14.
4. Emsley HH. Visual optics. 1st. London: Hatton Press;1948. p. 523.
5. London R, Wick BC. Changes in angle lambda during growth: theory and clinical applications. Am J Optom Physiol Opt. 1982; 59:568–72.
6. Le Grand Y, El Hage SG. Physiological optics. 1st. Berlin: Springer;1980. p. 72.
7. Hashemi H, KhabazKhoob M, Yazdani K. . Distribution of an-gle kappa measurements with Orbscan II in a population-based survey. J Refract Surg. 2010; 26:966–71.
8. Choi SR, Kim US. The correlation between angle kappa and ocular biometry in Koreans. Korean J Ophthalmol. 2013; 27:421–4.
9. Hwang BN, Son MH, Won IG. Angle kappa measurement with slit lamp biomicroscope. J Korean Ophthalmol Soc. 2002; 43:2005–9.
10. DeRespinis PA, Naidu E, Brodie SE. Calibration of Hirschberg test photographs under clinical conditions. Ophthalmology. 1989; 96:944–9.
11. Schaeffel F. Kappa and Hirschberg ratio measured with an auto-mated video gaze tracker. Optom Vis Sci. 2002; 79:329–34.
12. Jain R, Grewal S. Pentacam: principle and clinical applications. J Curr Glaucoma Pract. 2009; 3:20–32.
13. Riddell PM, Hainline L, Abramov I. Calibration of the Hirschberg test in human infants. Invest Ophthalmol Vis Sci. 1994; 35:538–43.
Figure 1.
Schematic representation of the components of angle lambda. (A) Definition of angle lambda. (B) Schematic diagram showing the geometrical model used to determine the angle Lambda and Calculation of angle lambda. (C) Angle Kappa, as measured clinically (AXF). (D) Angle lambda and angle kappa. F = fixation point; λ = angle lambda; E = center of Entrance of pupil; C = center of curvature of the cornea; A = end of extension line of the pupillary axis; X = point of the cornea that lies in the central pupil-lary line; K = angle kappa; N =nodal point.
Figure 2.
Clinical correlations of angle lambda and clinical factors. Exotropia (A), refractive errors (B), age (C), and axial length (D).
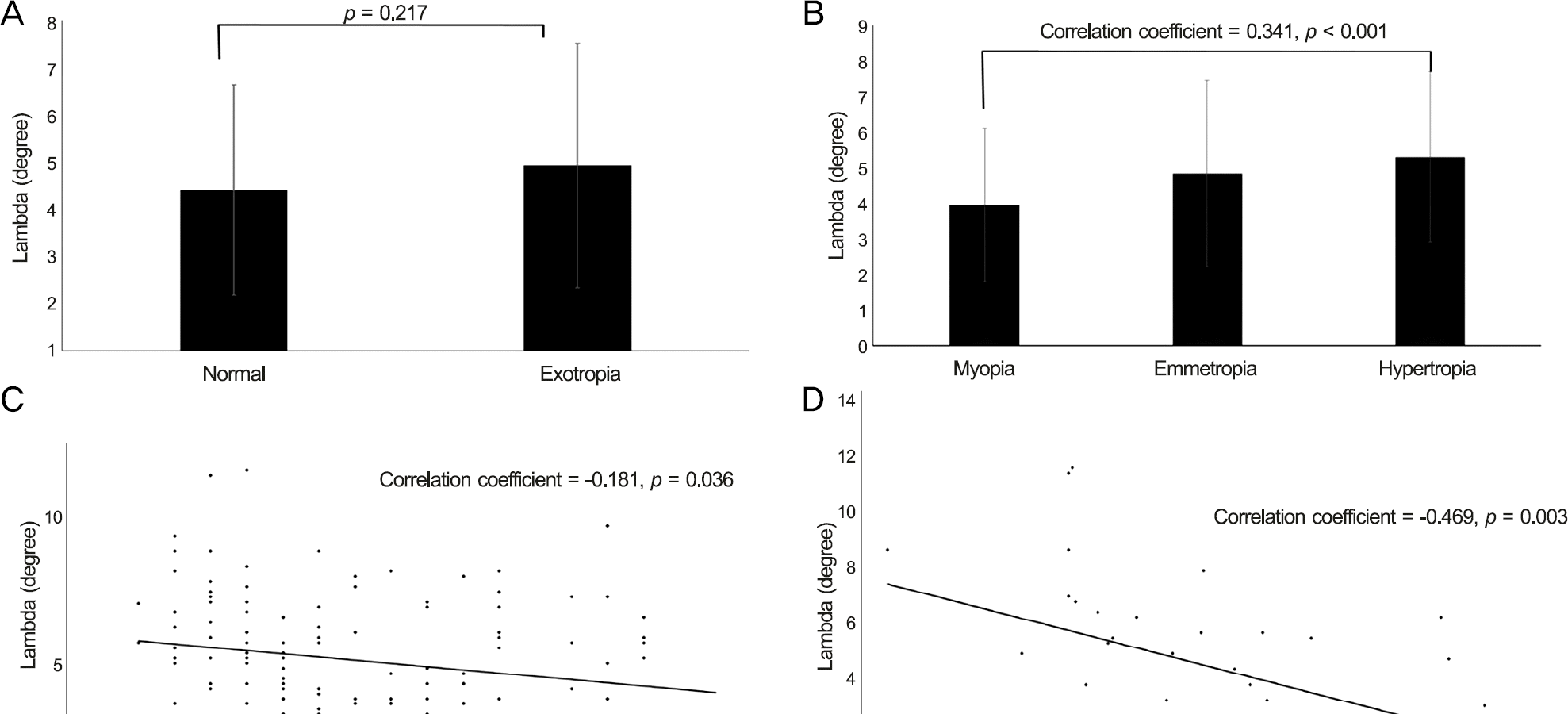
Table 1.
Demographic characteristics of the study groups
XML Download